Hypersonic Similarity

The linear theory is not valid at high supersonic Mach numbers, since:

^ 1 is true only for supersonic flow, and ^ 1 for hypersonic flow.

Even slender bodies produce large disturbances in hypersonic flow. The original nonlinear equations have to be used for such flows. So, mathematically hypersonic flow is similar to transonic flow. In supersonic flow, slender bodies produce weak shocks and so these can be considered as Mach lines.[17] But in hypersonic flow, even slender bodies produce strong shocks and, therefore, in hypersonic flow we can no more deal with Mach lines and must deal with the actual shock waves. At high Mach numbers, the Mach angle a may be of the same order or less than the maximum deflection angle в of the body.

From these considerations, the similarity rule can be obtained for hypersonic flow. The Mach angle a is given by the relation:

sin Ц =

For the present case of flow shown in Figure 9.14:

1

sin а яь a = —— < в,

where в is the half angle of the wedge in the figure, that is, for hypersonic flow:

Мтов > 1. (9.99)

But in hypersonic flows even for small disturbances, there are shock lines and the angle of shock is always less than the angle of Mach line. Therefore, in reality the inequality in Equation (9.99), obtained with the approximation that Mach angle a is of the same order or less than the flow turning angle в, has to be modified since the shock angle is always less than a. In other words, it can be stated that Мтов is greater than some quantity K, whose numerical value can be less than unity also.

K = И^в > 0.5
K = ме,

where K is called the Hypersonic similarity parameter.

Example 9.2

For в = 10° (^ 0.174 radian), Mx = 4; the hypersonic similarity parameter K = М^в = 0.7. For в = 20° and M, x) = 2:

K = М^в ^ 0.7.

That is, for a wedge with half-angle 20°, Mx = 2 should be considered as hypersonic. This implies that M > 5 for hypersonic flow is only a crude limit. For в = 5° and MOT = 8:

K = M^в ^ 0.7.

Thus, a wedge with half-angle 5° in a flow with Mx = 8 produces shocks as strong as a wedge with half-angle 20° in a flow with MOT = 2.

Also, by Equation (9.98):

1 t 2a

» = *0 ± a = 2 tl1 ± – cl’ (!U02)

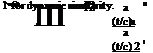Whenever M^в is the same for a number of bodies, the flow about them will be dynamically similar, that is, to investigate the hypersonic flow about a wedge with half-angle 5° and Mx = 8, we can use a supersonic tunnel with Mx = 2 and в = 20°. This is of paramount importance in testing; of course the two bodies should be affinely related (geometrically similar). Consider two models, 1 and 2:

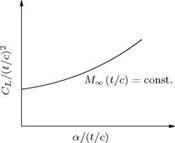
Figure 9.15 Variation of CL/(t/c)2 with a/(t/c).

This condition for dynamic similarity will be satisfied only when:

M<x>1 ^ c j = M<x,2 (c) •

That is, these two conditions should be satisfied for dynamic similarity, when there is geometric similarity:

c c = ( LcY M?"»( O-ttc)- (9Л03)

The total lift and drag coefficients are given by:

Cl =( C )! F,(«„( L).JL ) <9-104>

C„ = ( C )’ F, (m„ ( C ).tL ]. (9105)

Equations (9.103)—(9.105) give the functional dependence of various aerodynamic coefficients for hy­personic flow.

A plot like the one shown in Figure 9.15 gives the correct representation of the different parameters. This similarity rule is valid for axially symmetric bodies like rockets and missiles, also.

The transonic and hypersonic similarity rules discussed here are just a few glimpses, highlighting some of the vital features associated with them. Those who are looking for a deeper understanding of these problems should consult standard books on these topics.