As was mentioned above, take-off is a potentially hazardous operation and consequently steps must be taken to make the risk of accident acceptably low. The first thing to note in this regard is that all machines fail at some time or other and aero engines are no exception to this rule. During the take-off the
|
Fig. 13.2 Take-off
An F-18 in take-off configuration with flaps and leading-edge devices partially deployed
|
engines are working particularly hard and it is necessary to analyse the effect of likely failure at all stages in the take-off procedure and to ensure that enough runway is available to abort the take-off should this be necessary.
Multiple engined aircraft have the obvious advantage that it is possible to design so that failure of one engine can be tolerated and the take-off continued on the remaining engine or engines. This advantage is not gained without some complication, however. If an outboard engine were to fail then, because the other engines are operating near full thrust, a large yawing moment is produced which must be counteracted by the fin and rudder of the aircraft (Fig. 13.3). The low speed rudder authority required can lead to some quite large fin and rudder assemblies (Fig. 13.4).
Frequently this yawing moment is the factor which decides on the size of the fin and rudder for an aircraft, rather than any consideration of normal flight and manoeuvre. Another problem which may arise is due to the fact that the rudder authority depends on the air speed. Unless the speed is high enough then the rudder authoriy will not be great enough to cope with the ‘engine out’ case and the aircraft must not be ‘rotated’ for take-off until sufficient authority is
|
Fig. 13.3 Engine failure during take-off
Fin and rudder must be able to counteract yaw due to asymmetrical engine thrust
|
|
Fig. 13.4 Twin-engined aircraft designed for a low landing speed may require a very large fin to cope with the yawing moments produce on failure of one engine as on this D. H. Canada Dash-8
|
available. It may be this factor, rather than the aircraft stalling speed, which limits the take-off speed in a particular case.
During the take-off the pilot has to be aware of all factors such as the above, which may have safety implications. He will need to know exactly at which point along the runway that the ‘point of no return’ occurs where there will be insufficient remaining runway length to abort the take-off and bring the
aircraft to rest, as well as being aware of the point at which sufficient rudder control authority will be available to cope with the worst engine failure case envisaged for the type of aircraft.
A further complicating factor is that neither of these conditions depends solely on the type of aircraft and the length of runway from which it is operating. The aircraft take-off weight may vary between wide limits and the local weather will also affect the calculations. In general if the airfield is at high altitude, the air density will be reduced which will reduce the aerodynamic forces on the aircraft at a given speed. High ambient temperature will also reduce engine performance and change the calculations yet again. Head or tail winds will also alter the pilot’s calculations.
Thus critical conditions during the take-off have to be carefully evaluated before each flight, bearing such local factors in mind. Pilots therefore have a large amount of ‘homework’ to do before taking to the air!
Clearly the pilot’s workload during the take-off is high and a relatively straightforward method must be used to ensure that the safety requirements are met. Thus for a multi-engined aircraft a ‘decision’ speed, V1, is worked out for the particular take-off conditions. If an engine fails, or other failure occurs before this speed is reached then the pilot knows that it is possible to bring the aircraft safely to rest in the remaining runway length. If the speed is higher than V1 when the failure occurs it will be better to continue with the take-off and land later.
The important points in the take-off of a typical jet aircraft are shown in Fig. 13.5. Following the decision speed, V1, the aircraft continues to accelerate until the ‘rotation’ speed, VR, is reached at which point the nose is lifted. The aircraft takes off and starts the initial climb out at the safe take-off speed V2. As we have seen above, this speed may be determined by a number of factors. Which particular factor determines the rotation speed depends on the aircraft design and the circumstances of its operation. Firstly it may be determined simply by the requirment to have an adequate safety margin above the stalling speed. Secondly the speed may have to be somewhat above this value because, for example, the angle of incidence obtainable on the ground may be limited by tail clearance to a value well below the stalling incidence. Finally the speed may be dictated by the rudder control requirement which accompanies engine failure.
35 ft
V3
Climb
Fig. 13.5 Take-off speeds
Critical points of the take-off are defined by speeds which are easily monitored by the pilot
The take-off manoeuvre is regarded as complete when the aircraft passes over the ‘screen’ height of 35 ft for a jet transport. The speed at this point is known as V3. After the screen height has been reached, the pilot has to comply with noise requirements in the subsequent climb-out. The aircraft must also be designed to climb safely and return for landing following engine failure.
The relatively high take-off speed for jet aircraft might make the operation appear more dangerous than for a piston-engined type, but the probability of engine failure is much lower for the jet than for a piston engine working at its maximum rating.












 Speed (m/s)
Speed (m/s)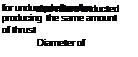
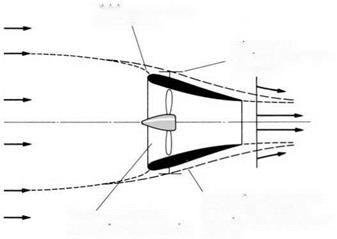 Fig. 6.13 Ducted fan at low speed
Fig. 6.13 Ducted fan at low speed