When air enters the intake of a jet engine, its speed is reduced, and the pressure rises correspondingly. This ram compression effect means that as the aircraft speed rises, the compressor becomes less and less necessary. At Mach numbers in excess of about 3 (three times the speed of sound), efficient propulsion can be obtained with no compressor at all. Elimination of the compressor means that the turbine is also unnecessary. All that is required is a suitably shaped duct with a combustion chamber. This extremely simple form of jet propulsion is known as a ramjet.
The basic principle of the ramjet is illustrated in Fig. 6.37. The thrust force is produced mainly by a high pressure acting on the interior walls of the intake. For efficient operation at high Mach numbers, a more complicated intake geometry is required; similar to the types used for the supersonic turbo-jet propulsion, as described above.
The problem with ramjets is that they are inefficient below a Mach number of about 3, and will not work at all if there is no forward motion. Some other form of propulsion is required to provide the initial acceleration to high speed. In the case of missiles, an initial booster rocket is normally used. In the early postwar era, the French Leduc company produced a number of ramjet-propelled experimental aircraft which flew successfully (Fig. 6.38). They were normally flight-launched from a mother aircraft, and landed as gliders.
At very high Mach numbers, it becomes necessary to have supersonic flow in the combustion area. This is known as a scramjet (supersonic combusting ramjet) propulsion. Conventional combustion systems that simply involve adding fuels such as kerosene to the air flow cannot be used, as the flame would not propagate as fast as the flow, and it would thus simply blow out. The unmanned X-43A hypersonic research aircraft powered by a scramjet engine is described in Chapter 8, and shown in Fig. 8.24.
|
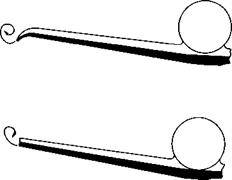
Fig. 6.38 The French Leduc 010 experimental ramjet aircraft of 1946
The aircraft was air-launched from its mounting above a modified airliner, and landed as a glider. The pilot lay in a prone position in the nose cone, and must have had great courage. A later development the Leduc 022 of 1954 achieved supersonic flight
|
The dual-mode turbo-ramjet
As an alternative to air launching or a booster engine, some form of dual – or multi-mode propulsion may be used. One approach is to use a turbo-jet engine inside a ramjet duct, as illustrated in Fig. 6.39. At low speeds, the engine performs as a conventional turbo-jet. At high Mach numbers, however, some or all of the air may be by-passed around the main core engine and used in an afterburner to produce ramjet propulsion.
The advantage of this arrangement over a conventional turbo-jet is that the ramjet becomes more efficient at high Mach numbers, because the energy degradation in the turbine and compressor is eliminated. The SR-71 shown in Fig. 6.40 uses a form of turbo-ramjet propulsion.
Unfortunately, at the Mach number where ramjet propulsion becomes efficient, kinetic heating effects render conventional aluminium alloys and construction techniques unsuitable. Very few aircraft with a Mach 3 capability have been built, and most of these have been experimental or research vehicles. The SR-71 reconnaissance aircraft shown in Fig. 6.40 is a rare example of a production machine with Mach 3+ capability. This has now been withdrawn from active service.
Nozzle in
convergent
Primary n. – divergent
flow Coreengine configuration
Subsonic flow
Fig. 6.39 Schematic arrangement of a turbo-ramjet
At high supersonic speeds, the primary flow by-passes the core turbo-jet, and the afterburner is used to provide ramjet propulsion
The central spike moves in and out axially to match the intake geometry to the flight conditions
For efficient operation, the spike shock wave should just strike the intake rim.
The spike is also moved when ‘starting’ the intake shock system
(a) Ramjet mode at high supersonic speed (b) Turbo-jet mode at subsonic speed