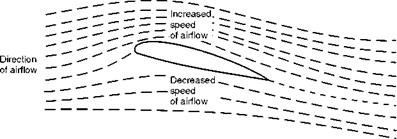
Lift, drag and pitching moment of an aerofoil
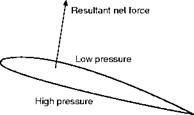
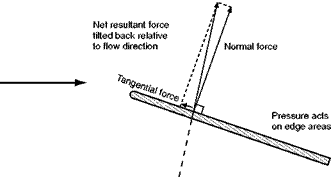
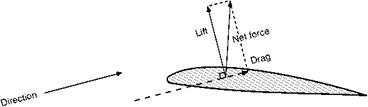
Now the ultimate object of the aerofoil is to obtain the lift necessary to keep the aeroplane in the air; in order to obtain this lift it must be propelled through the air at a definite velocity and it must be set at a definite angle of attack to the flow of air past it. We have already discovered that we cannot obtain a purely vertical force on the aerofoil; in other words, we can only obtain lift at the expense of a certain amount of drag. The latter is a necessary evil, and it must be reduced to the minimum so as to reduce the power required to pull the aerofoil through the air, or alternatively to increase the velocity which we can obtain from a given engine power. Our next task, therefore, is to investigate how much lift and how much drag we shall obtain from different shaped aerofoils at various angles of attack and at various velocities. The task is one of appalling magnitude; there is no limit to the number of aerofoil shapes which we might test, and in spite of thousands of experiments carried out in wind tunnels and by full-scale tests in the air, it is still impossible to say that we have discovered the best-shaped aerofoil for any particular purpose. However, modern theoretical methods make it possible to predict the behaviour of aerofoil sections. Such methods can even be used to design aerofoils to give specified characteristics.
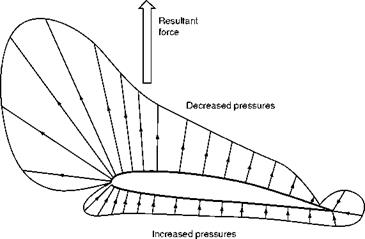
In wind-tunnel work it is the usual practice to measure lift and drag separately, rather than to measure the total resultant force and then split it up into two components. The aerofoil is set at various angles of attack to the airflow, and the lift, drag and pitching moment are measured on a balance.
The results of the experiments show that within certain limitations the lift, drag and pitching moment of an aerofoil depend on –
(a) The shape of the aerofoil.
(b) The plan area of the aerofoil.
(c) The square of the velocity.
(d) The density of the air.
Notice the similarity of these conclusions to those obtained when measuring drag, and in all cases there are similar limitations to the conclusions arrived at.
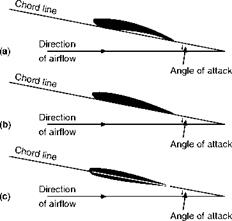
The reader should notice that whereas when measuring drag we considered the frontal area of the body concerned, on aerofoils we take the plan area. This is more convenient because the main force with which we are concerned, i. e. the lift, is at right angles to the direction of motion and very nearly at right angles to the aerofoils themselves, and therefore this force will depend on the plan area rather than the front elevation. The actual plan area will alter as the angle of attack is changed and therefore it is more convenient to refer results to the maximum plan area (the area projected on the plane of the chord), so that the area will remain constant whatever the angle of attack may be. Now we use the symbol S, for the plan area of a wing, to replace the frontal areas, which we used when considering drag alone in the previous chapter.
In so far as the above conclusions are true, we can express them as formulae in the forms –
|
Lift = |
cL- |
IpV2. |
S or CL. q |
. S |
|
Drag = |
Co- |
1 ру1 ‘ |
, S or CD. q |
. s |
|
Pitching moment = |
CM |
• IpV2 |
• Sc or CM. |
q. Sc |
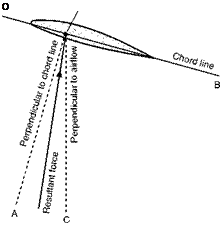
Since the pitching moment is a moment, i. e. a force X distance, and since IpV2. S represents a force, it is necessary to introduce a length into the equation – this is in the form of the chord, c, measured in metres.
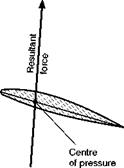
The pitching moment is positive when it tends to push the nose upwards, negative when the nose tends to go downwards.
The symbols CL, CD and CM are called the lift coefficient, drag coefficient and pitching moment coefficient of the aerofoil respectively; they depend on the shape of the aerofoil, and they alter with changes in the angle of attack. The air density is represented by p in kilograms per cubic metre, S is the plan area of the wing in square metres, У is the air speed, in metres per second, c the chord of the aerofoil in metres; the method of writing the formulae in terms of IpV2, or q, has already been explained in Chapter 2.
Aerofoil characteristics
The easiest way of setting out the results of experiments on aerofoil sections is to draw curves showing how –
(a) the lift coefficient,
(b) the drag coefficient,
(c) the ratio of lift to drag, and
(d) the position of the centre of pressure, or the pitching moment coefficient,
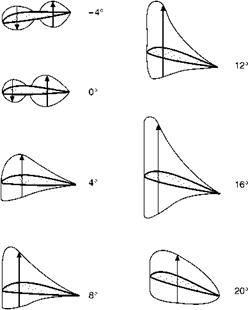
alter as the angle of attack is increased over the ordinary angles of flight.
Typical graphs are shown in Figs 3.13, 3.15, 3.16 and 3.17. These do not refer to any particular aerofoil; they are intended merely to show the type of curves obtained for an ordinary general purpose aerofoil.
In Appendix 1 at the end of the book, tables are given showing the values of CL, CD, L/D, position of the centre of pressure, and CM, for a few well – known aerofoil sections. The reader is advised to plot the graphs for these sections, and to compare them with one another (see example Nos. 98 to 101 in Appendix 3). In this way the reader will understand much more clearly the arguments followed in the remaining portion of this chapter.
It is much more satisfactory to plot the coefficients of lift, drag and pitching moment rather than the total lift, drag and pitching moment, because the coefficients are practically independent of the air density, the scale of the aerofoil and the velocity used in the experiment, whereas the total lift, drag and moment depend on the actual conditions at the time of the experiment. In other words, suppose we take a particular aerofoil section and test it on different scales at different velocities in various wind tunnels throughout the world, and also full-scale in actual flight, we should in each case obtain the same curves showing how the coefficients change with angle of attack.
It must be admitted that, in practice, the curves obtained from these various experiments do not exactly coincide; this is because the theories which have led us to adopt the formula lift = CL. ypV2 . S are not exactly true for very much the same reasons as those we mentioned when dealing with drag – for instance, scale effect and the interference of wind-tunnel walls. As a result of the large number of experiments which have been performed, it is possible to make allowances for these errors and so obtain good accuracy whatever the conditions of the experiment.
Now let us look at the curves to see what they mean, for a graph which is properly understood can convey a great deal of information in a compact and practical form.













 of
of