Linear quadratic Gaussian (LQG) controllers add to the linear quadratic (LQ) designs random disturbances and measurement errors. LQG designs are discussed at length in a 1986 text and a 1993 IEEE paper by Professor Robert F. Stengel. The form taken by
|

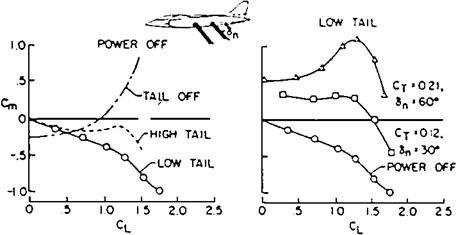
Figure 20.8 Various control system forms that can be represented with the structured linear quadratic regulator(LQG)method. (FromStengel, IEEE Trans. onSystems, Man, andCybernetics, © 1993 IEEE)
|
the discrete-time LQG optimal controller is
uk = CFyk* – CBxk,
where yk * is the desired value of an output vector and xk is the Kalman filter state estimate.
The LQG design approach is very flexible because of the number of parameters that can be chosen arbitrarily. At one extreme, a scalar one-input, one-output design can be produced. Measurement and control redundancies can be represented if measurement and control vector sizes exceed that of the state vector. Also, integral compensation and explicit model-following structures can be produced (Figure 20.8).
LQG designs are among the most advanced to be in use by stability-augmentation engineers, as this is written. Even more advanced control concepts continue to pour out of university and other research centers. The same 1993 paper by Stengel cited above provides a good survey of advanced control concepts, including expert systems, neural networks, and intentionally nonlinear controls.
20.3 Failed Applications of Optimal Control
The failure of optimal control methods to produce a satisfactory flight control system for the Grumman X-29A airplane was noted in Sec. 14. This failure is by no means an isolated event. Additional instances can be found in which optimal control methods in the hands of experienced engineers have failed to produce safe and satisfactory flight control systems. What has gone wrong? Several experts who have witnessed these failures discuss the problem:
Phillip R. Chandler and David W. Potts (1983), U. S. Air Force Flight Dynamics Laboratory “[T]he infinite bandwidth constant compensation elements which are required [for LQR] violate the very heart of the feedback problem. . . . LQR therefore is an elegant mathematical solution to a nonengineering problem SVT (Singular Value Theory) [Doyle, 1979] is a very crude
method of coping with uncertainty in the LQR or LQG procedure. It makes assumptions that are not valid for flight control_______________________________________ LQR with all its ramifica
tions and refinements is totally unsuited for the flight control servomechanism problem.”
John C. Gibson (2000), formerly with English/Electric/British Aerospace
“[Robert J.] Woodcock told me that there have been several missile and aircraft projects in serious trouble due to the use of such [LQG] methods_______________________________________ While op
timization methods are continually being improved, they cannot yet (and may never) guarantee a safe and satisfactory FCS [flight control system] design without the strictest guidance and detailed physical understanding of experienced control and handling qualities engineers. This is true for highly advanced and demanding types of aircraft. Every signal path must be clearly visible and easily related to specific aerodynamic or inertial characteristics of the airframe. In simple aircraft without complexity, there is no advantage over straightforward engineering methods anyway.”
Michael V. Cook (1999, 2000), Senior Lecturer, Cranfield University “There exists an enormous wealth of published material describing the application of so-called, ‘modern control methods’ to the design of flight control systems for piloted aeroplanes. It is also evident, with the exception of a very small number of recent applications, that there is a conspicuous lack of enthusiasm on the part of the airframe manufacturers to adopt this design technology, especially for the design of command and stability augmentation systems for piloted airplanes. Having an industrial background I am well aware of the many reasons why modern control has not been taken onboard seriously by the manufacturers – academic control specialists don’t share my view, and in many cases probably don’t even understand it!… I know that my views are shared by the control
people in——- who, in private are not at all complimentary about the academic
control specialists in the UK. I am also aware that the Boeing view is similar to
that of—— . I’ve seen some appallingly bad control systems design theses (not
from Cranfield).”
Steven Osder (2000), Osder Associates, Arizona “We [Osder and Dunstan Graham] used to lament the absurdity of papers [on robustness theory] that were filling the journals and we amused each other by citing specific examples of such departures from reason and logic_____________________ At the [Boeing] helicopter com
pany, we took each of those University of – [robust flight control] designs and tested them against more complete [nonlinear] models of the [Apache] aircraft.
In every case, these robust flight control designs always fell out of the sky. In one case [which used eigenstructure assignment], even testing against a linear model, but with only a 10 percent variation in a single B [control] matrix term, our simulations resulted in a crash.”
Duane T. McRuer (2001), Chairman, Systems Technology, Inc. “At STI we have spent an enormous amount of time and effort searching for ways to make optimal control practical – at least 20 major reports and papers, with some tremendously capable folk (e. g., Dick Whitbeck, Greg Hofmann, Bob Stapleford, Peter Thompson, et al.). Our focus has been on finding performance indices, special schemes, etc., to make optimal control solutions jibe with good
design practice__ We have just never been happy with the results for stability
augmentation design.”
In the light of the foregoing comments, a design case (Ward, 1996) in which an LQG design for a pitch stability augmentation system was used only as a guideline for a more conventional approach suggests a reasonable use for optimal control techniques. The concept of using LQR optimal control synthesis as a guide or in conjunction with classical methods is also developed by Blight (1996). Blight also comments that LQR methods should be used only on “control problems that actually require modern multivariable methods for their solution.” For example, Blight recommends ordinary gain scheduling instead of attempting to design a single robust linear control law for all flight conditions.