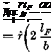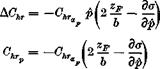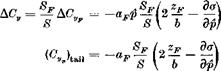Category Dynamics of. Atmospheric Flight

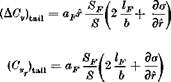The only contribution to Cy that is normally important is that of the tail. From the angle-of-attack change we find the incremental Cy to be

THE DERIVATIVE C,

bt

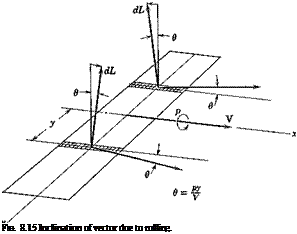
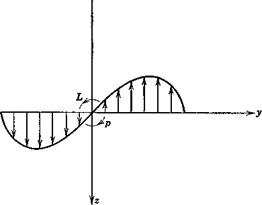
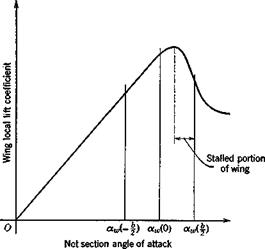
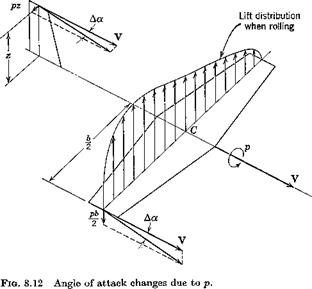
This is another important cross derivative; the rolling moment due to yawing. The increase in lift on the left wing, and the decrease on the right wing combine to produce a positive rolling moment proportional to the original lift coefficient CL. Hence this derivative is largest at low speed. Aspect ratio, taper ratio, and sweepback are all important parameters.

When the vertical tail is large, its contribution may be significant. A formula for it can be derived in the same way as for the previous tail contributions, with the result

THE DERIVATIVE C„

,lr

СПг is the damping-in-yaw derivative, and is always negative. The body adds a negligible amount to СПг except when it is very large. The important contributions for airplanes are those of the wing and tail. The increases in both the profile and induced drag on the left wing and the decreases on the right wing give a negative yawing moment and hence a resistance to the motion. The magnitude of the effect depends on the aspect ratio, taper ratio, and sweepback. For extremely large sweepback, of the order of 60°, the yawing moment associated with the induced drag may be positive: i. e. produce a reduction in the damping.

The side force on the tail also provides a negative yawing moment. The calculation is similar to that for the preceding tail contributions, with the result

2f + d?) (8’7;4)

Just as with Cm, there is a damping-in-yaw provided by the propulsive jet on jet and rocket vehicles. The calculation of ДGm applies exactly to this case as well if M be replaced by N, and q by r. The result is the same as (7.9,20 and 22), i. e.

Д Nr = -2 т’Ц

АСПг = -4СТ? гЩ (8.7,5)

V} c“

When an airplane has a rate of yaw r superimposed on the forward motion V, its velocity field is altered significantly. This is illustrated for the wing

and vertical tail in Fig. 8.16. The situation on the wing is clearly very complicated when it has much sweepback. The main feature however, is that the velocity of the f chord line normal to itself is increased by the yawing on the left-hand side, and decreased on the right side. The aero­dynamic forces at each section (lift, drag, moment) are therefore increased on the left-hand side, and decreased on the right-hand side. As in the case

of the rolling wing, the uasymmetrical lift distribution leads to an unsym­metrical trailing vortex sheet, and hence a sidewash at the tail. The incre­mental tail angle of attack is then

V dr

This derivative gives the change of aileron hinge moment due to rolling. It occurs because of the change in wing angle of attack at the ailerons, and because Ghx of the ailerons is usually nonzero. Let ya be the spanwise co­ordinate of the right hand mid-aileron section. Then the approximate change in angle of attack at the right hand aileron is

A

V

THE DERIVATIVE CM

The change in vertical-tail angle of attack brought about by p produces a change in the rudder hinge moment. This is given by

When ChTx is negative, as for a simple flap control, then a positive roll produces a positive rudder hinge moment.

ftp

The yawing moment produced by the rolling motion is one of the so called cross derivatives. It is the existence of these cross derivatives that causes the rolling and yawing motions to be so closely coupled. The wing and tail both contribute to Gn.

The wing contribution is in two parts. The first comes from the change in

profile drag associated with the change in wing angle of attack. The wing a is increased on the right-hand side and decreased on the left-hand side. These changes will normally be accompanied by an increase in profile drag on the right side, and a decrease on the left side, combining to produce a positive (nose-right) yawing moment. The second wing effect is associated with the fore-and-aft inclination of the lift vector which is caused by the rolling in subsonic flight and in supersonic flight when the leading edge is subsonic. Its existence depends on the leading edge suction. The physical situation is illustrated in Fig. 8.15. The directions of motion of two typical

wing elements are shown inclined by the angles ±0 = py/V from the direction of the vector Y. Since the local lift is perpendicular to the local relative wind, then the lift vector on the right half of the wing is inclined forward, and that on the left half backward. The result is a negative yawing couple, proportional to the product CLp. If the wing leading edges are supersonic, then the leading-edge suction is not present, and the local force remains normal to the surface. The increased angle of attack on the right side causes an increase in this normal force there, while the opposite happens on the left side. The result is a positive yawing couple proportional to p.

The tail contribution to G„ is easily found from the tail side force given previously (8.6,2). The incremental Gn is given by

where lF is the distance shown in Fig. 8.2. Therefore

where Vv is the vertical-tail volume ratio.

Сг is known as the damping-in-roll derivative. It expresses the resistance of the airplane to rolling. Except in unusual circumstances, only the wing contributes significantly to this derivative. As can be seen from Fig. 8.12, the angle of attack due top varies linearly across the span, from the value pb/2V at the right wing tip to —pbj’2 V at the left tip. This antisymmetric a dis­tribution produces an antisymmetric increment in the lift distribution as shown in Fig. 8.13. In the linear range this is superimposed on the symmetric

lift distribution associated with the wing angle of attack in undisturbed flight. The large rolling moment L produced by this lift distribution is proportional to the tip angle of attack p, and <7^ is a negative constant, so long as the local angle of attack remains below the local stalling angle.

If the wing angle of attack at the center line, a„(0), is large, then the incremental value due to p may take some sections of the wing beyond the stalling angle, as shown in Fig. 8.14. [Actually, for finite span wings, there is

an additional induced angle of attack distribution ац(у) due to the vortex wake that modifies the net sectional value still further. We neglect this correction here in the interest of making the main point.] When this happens GtJ) is reduced in magnitude from the linear value and if ocw(0) is large enough, will even change sign. When this happens, the wing will autorotate, the main characteristic of spinning flight.

The side force due to rolling is often negligible. When it is not, the con­tributions that need to be considered are those from the wingf and from the vertical tail. The vertical-tail effect may be estimated in the light of its angle-of-attack change (Fig. 8.12) as follows. Let the mean change in a. F (Fig. 8.2) due to the rolling velocity be

V dp

where zp is an appropriate mean height of the fin. Introducing the

■f For the effect of the wing at low speeds, see ref. (8.4).

Aop=-^2|?-|^ (8.6,1)

The incremental side-force coefficient on the fin is obtained from Aa^.,

A 0Vr = aF &<xF = – aFp[ 2 (§-6,2)

where aF is the lift-curve slope of the vertical tail. The incremental side force on the airplane is then given by

When an airplane rolls with angular velocity p about its x axis in the reference state (the flight direction for wind axes), its motion is instantaneously like that of a screw. This motion affects the airflow (local angle of attack) at all stations of the wing and tail surfaces. This is illustrated in Pig. 8.12 for two points: a wing tip and the fin tip. It should be noted that the non-dimensional rate of roll, p = p&/2F is, for small p, the angle (in radians) of the helix traced by the wing tip. These angle-of-attack changes bring about alterations in the aerodynamic load distribution over the surfaces, and thereby introduce perturbations in the forces and moments. The change in the wing load distribution also causes a modification to the trailing vortex sheet. The vorticity distribution in it is no longer symmetrical about the x axis, and a sidewash (positive, i. e. to the right) is induced at a vertical tail conventionally placed. This further modifies the angle-of-attack distribution on the vertical – tail surface. This sidewash due to rolling is characterized hy the derivative
да/dp. It has been studied theoretically and experimentally by Michael (ref. 8.1), who has shown its importance in relation to correct estimation of the tail contributions to the rolling derivatives. Finally, the helical motion of the wing produces a trailing vortex sheet which is not flat, but helical. For the small rates of roll admissible in a linear theory, this effect may be neglected with respect to both wing and tail forces.

The sideslipping airplane gives rise to a side force on the vertical tail as explained in Sec. 8.1. When the aerodynamic center of the vertical surface is appreciably offset from the rolling axis (Fig. 8.11) then this force may produce a significant rolling moment. From (8.1,2 and 3) with bT = 0 this

P + o) — V2Sfzf

It

A 0, = «,(-j5 + <r)^

<•**>

THE DERIVATIVE C

“P

This is the yaw stiffness derivative, already treated in detail in Sec. 8.1.

THE DERIVATIVE CAr

This derivative gives the rudder hinge moment due to sideslip. It is analogous to the elevator hinge moment due to angle of attack. It is given

ЪУ д£м dotp

dp hr*f эр

where is the appropriate coefficient—see (6.5,1). By using (8.1,1) we get

(8.5,4)

Wing sweep is a major parameter affecting Gt. Consider the swept yawed wing illustrated in Fig. 8.10. According to simple sweep theory it is the velocity Vn normal to a wing reference line (J chord line for subsonic, l. e. for super­sonic) that determines the lift. It follows obviously that the lift is greater

on the right half of the wing shown than on the left half, and hence that there is a negative rolling moment. The rolling moment would be expected for small (1 to be proportional to

tfiUJVWM – (K2)ien = 0хГ*[сов* (A — P) — cos2 (Л + /?)]

= 20 L(3 Vі sin 2Л

The proportionality with GL and (3 is correct, but the sin 2Л factor is not a good approximation to the variation with Л. The result is a oc CL, that can be calculated by the methods of linear wing theory.

The flow field of the body interacts with the wing in such a way as to modify its dihedral effect. To illustrate this, consider a long cylindrical body, of circular cross section, yawed with respect to the main stream. Consider only the cross-flow component of the stream, of magnitude F/S, and the flow pattern which it produces about the body. This is illustrated in Fig. 8.9. It

is clearly seen that the body induces vertical velocities which, when combined with the mainstream velocity, alter the local angle of attack of the wing. When the wing is at the top of the body (high-wing), then the angle-of-attack distribution is such as to produce a negative rolling moment: i. e. the dehedral effect is enhanced. Conversely, when the airplane has a low wing, the dihedral effect is diminished by the fuselage interference. The magnitude of the effect is dependent upon the fuselage length ahead of the wing, its cross-section shape, and the planform and location of the wing. Generally, this explains why high-wing airplanes usually have less wing dihedral than low-wing airplanes.