Category Dynamics of Flight

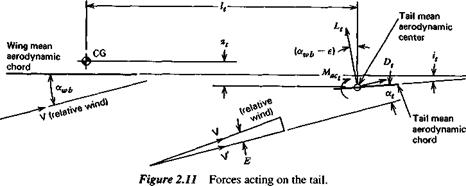
The moment provided by a propulsive system is in two parts: (1) that coming from the forces acting on the unit itself, for example, the thrust and in-plane force acting on a propeller, and (2) that coming from the interaction of the propulsive slipstream with the other parts of the airplane. These are discussed in more detail in Sec. 3.4. We assume that the interference part is included in the moments already given for the wing, body, and tail, and denote by Cmp the remaining moment from the propulsion units.

2.2 Total Pitching Moment and Neutral Point

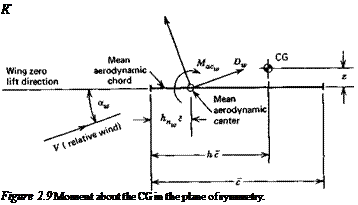
On summing the first of (2.2,4) and (2.2,13) making use of (2.2,6) and adding the contribution Cmp for the propulsive system, we obtain the total pitching moment about the CG

Cm = Gmo<,B, k + CL(h – hnJ – VHCL, + (2.3,1)

It is worthwhile repeating that no assumptions about thrust, compressibility, or aero – elastic effects have been made in respect of (2.3,1). The pitch stiffness ( —CmJ is now obtained from (2.3,1). Recall that the mean aerodynamic centers of the wing-body combination and of the tail are fixed points, so that

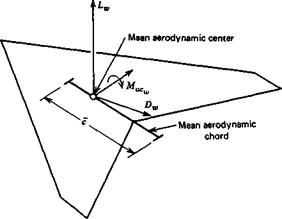
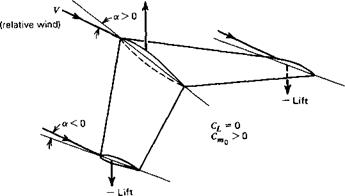
If a true mean aerodynamic center in the classical sense exists, then dCmacJda is zero and

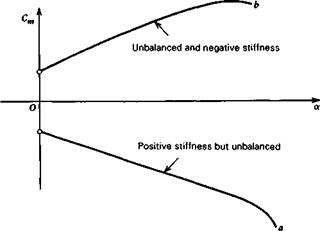
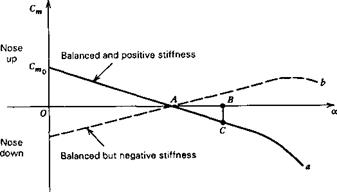
Cma as given by (2.3,2) or (2.3,3) depends linearly on the CG position, h. Since CI y is usually large, the magnitude and sign of Cma depend strongly on h. This is the basis of the statement in Sec. 2.2 that Cma can always be made negative by a suitable choice of h. The CG position hn for which Cma is zero is of particular significance, since this represents a boundary between positive and negative pitch stiffness. In this book we define hn as the neutral point, NP. It has the same significance for the vehi­cle as a whole as does the mean aerodynamic center for a wing alone, and indeed the term vehicle aerodynamic center is an acceptable alternative to “neutral point.”

The location of the NP is readily calculated from (2.3,2) by setting the left-hand side to zero leading to

Substitution of (2.3,4) back into (2.3,2) simplifies the latter to

Cm„ = CLa(h ~ hn) (2.3,5)

which is valid whether and Cm vary with a or not. Equation (2.3,5) clearly

provides an excellent way of finding hn from test results, that is from measurements of Cma and CL y. The difference between the CG position and the NP is sometimes called the static margin,

Kn = (hn – h) (2.3,6)

Since the criterion to be satisfied is Cnia < 0, that is, positive pitch stiffness, then we see that we must have h < hn, or Kn > 0. In other words the CG must be forward of the NP. The farther forward the CG the greater is Kn, and in the sense of “static stability” the more stable the vehicle.

The neutral point has sometimes been defined as the CG location at which the derivative dCJdCL = 0. When this definition is applied to the gliding flight of a rigid
airplane at low Mach number, the neutral point obtained is identical with that defined in this book. This is so because under these restricted conditions C, is a unique func­tion of a, and dCJdCL = (dCJda)/(dCL/da). Then dCJdCL and dCJda are simul­taneously zero. In general, however, Cm and CL are both functions of several vari­ables, as pointed out at the beginning of Sec. 2.2. For fixed values of Se and h, and neglecting Reynolds number effects (these are usually very small), we may write

CL = f(a, M, C-n hPV2), Cm = g(a, M, CT, pV2) (2.3,7)

where CT is the thrust coefficient, defined in Sec. 3.15.

Mathematically speaking, the derivative dCJdCL does not exist unless M, C7, and pV2 are functions of C,. When that is the case, then

dCm _ 3C,„ da ЭCm ЭМ dCm dCT dCm d(hpV2) ^ ^

dCL da dCL ЭМ dCL + dCT dCL + d{pV2) dCL (2’3’8)

Equation 2.3,8 has meaning only when a specific kind of flight is prescribed: e. g.,

horizontal unaccelerated flight, or rectilinear climbing flight at full throttle. When a condition of this kind is imposed, then M, CT, and the dynamic pressure are definite functions of CL, dCJdCL exists, and a neutral point may be calculated. The neutral point so found is not an index of stability with respect to angle of attack disturbances, and the question arises as to what it does relate to. It can be shown that it relates to the trim curves of the airplane. A plot of the elevator angle to trim versus speed will have a zero slope when dCJdCL is zero, and a negative slope when the CG lies aft of the neutral point so defined. As shown in Sec. 2.4, this reversal of slope indicates a tendency toward instability with respect to speed, but only a dynamic analysis can show whether or not the airplane is stable in this condition. There are cases when the application of the “trim-slope” criterion can be definitely misleading as to stability. One such is level unaccelerated flight, during which the throttle must be adjusted every time the flight speed or CL is altered.

It can be seen from the foregoing remarks that the “trim-slope” criterion for the neutral point does not lead to any definite and clear-cut conclusions, either about the stability with respect to angle of attack disturbances, or about the general static sta­bility involving both speed and angle of attack disturbances. It is mainly for this rea­son that the neutral point has been defined herein on the basis of dCJda.

The forces on an isolated tail are represented just like those on an isolated wing. When the tail is mounted on an airplane, however, important interferences occur. The most significant of these, and one that is usually predictable by aerodynamic theory, is a downward deflection of the flow at the tail caused by the wing. This is character­ized by the mean downwash angle e. Blanking of part of the tail by the body is a sec­ond effect, and a reduction of the relative wind when the tail lies in the wing wake is the third.

Figure 2.11 depicts the forces acting on the tail showing the relative wind vector of the airplane. V’ is the average or effective relative wind at the tail. The tail lift and drag forces are, respectively, perpendicular and parallel to V’. The reader should note

the tail angle /„ which must be positive as shown for equilibrium. This is sometimes referred to as longitudinal dihedral.

The contribution of the tail to the airplane lift, which by definition is perpendicu­lar to V, is

L, cos e — D, sin e

e is always a small angle, and we assume that Dte may be neglected compared with Lt. The contribution of the tail to the airplane lift then becomes simply L,. We intro­duce the symbol CLt to represent the lift coefficient of the tail, based on the airplane dynamic pressure |pV2 and the tail area S,.

L,

lpV2S,

The total lift of the airplane is or in coefficient form

Cl = Cu, + j CL, (2.2,6)

The reader should note that the lift coefficient of the tail is often based on the local dynamic pressure at the tail, which differs from |pV2 when the tail lies in the wing wake. This practice entails carrying the ratio V’/V in many subsequent equations. The definition employed here amounts to incorporating V’/V into the tail lift-curve slope a,. This quantity is in any event different from that for the isolated tail, owing to the interference effects previously noted. This circumstance is handled in various ways in the literature. Sometimes a tail efficiency factor 17, is introduced, the isolated tail lift slope being multiplied by 17,. In other treatments, 17, is used to represent (V’/V)2. In the convention adopted here, a, is the lift-curve slope of the tail, as measured in situ on the airplane, and based on the dynamic pressure pV2. This is the quantity that is directly obtained in a wind-tunnel test.

From Fig. 2.11 we find the pitching moment of the tail about the CG to be

M, = —l,[L, cos (awb – є) + D, sin (awh – є)]

– z,[D, cos (awh – e) – L, sin (awb – e)] + Mai. (2.2,7)

Experience has shown that in the majority of instances the dominant term in this equation is the first one, and that all others are negligible by comparison. Only this case will be dealt with here. The reader is left to extend the analysis to cases in which this approximation is not valid. With the above approximation, and that of small an­gles,

M, = – I A = —l, CL£pV2S,

Upon conversion to coefficient form, we obtain

The combination l, S,/Sc is the ratio of two volumes characteristic of the airplane’s

geometry. It is commonly called the “horizontal-tail volume ratio,” or more simply, the “tail volume.” It is denoted here by VH. Thus

cm, = – VHCL, (2.2,9)

Since the center of gravity is not a fixed point, but varies with the loading condi­tion and fuel consumption of the vehicle, VH in (2.2,9) is not a constant (although it does not vary much). It is a little more convenient to calculate the moment of the tail about a fixed point, the mean aerodynamic center of the wing-body combination, and to use this moment in the subsequent algebraic manipulations. Figure 2.12 shows the relevant relationships, and we define

– Is,

VH=~ (2.2,10)

cS

which leads to

V„=VH – j(h~hnJ (2.2,11)

The moment of the tail about the wing-body mean aerodynamic center is then [cf.

(2.2,9) ]

C„„ = ~V„CL, (2.2,12)

and its moment about the CG is, from substitution of (2.2,11) into (2.2,9)

Cm, = – VHCL, + CLt j(h – hnJ (2.2,13)

The influences of the body and nacelles are complex. A body alone in an airstream is subjected to aerodynamic forces. These, like those on the wing, may be represented over moderate ranges of angle of attack by lift and drag forces at an aerodynamic center, and a pitching couple independent of a. Also as for a wing alone, the lift-а re­lation is approximately linear. When the wing and body are put together, however, a simple superposition of the aerodynamic forces that act upon them separately does not give a correct result. Strong interference effects are usually present, the flow field of the wing affecting the forces on the body, and vice versa.

These interference flow fields are illustrated for subsonic flow in Fig. 2.10. Part (a) shows the pattern of induced velocity along the body that is caused by the wing vortex system. This induced flow produces a positive moment that increases with wing lift or a. Hence a positive (destabilizing) contribution to Cma results. Part (b) shows an effect of the body on the wing. When the body axis is at angle a to the stream, there is a cross-flow component V sin a. The body distorts this flow locally, leading to cross-flow components that can be of order 2У sin a at the wing-body in­tersection. There is a resulting change in the wing lift distribution.

The result of adding a body and nacelles to a wing may usually be interpreted as a shift (forward) of the mean aerodynamic center, an increase in the lift-curve slope, and a negative increment in Cmac. The equation that corresponds to (2.2,3) for a wing – body-nacelle combination is then of the same form as (2.2,3), but with different val­ues of the parameters. The subscript wb is used to denote these values.

~~ CmaCwh Сь„ь(Ь Ь-п„ь)

= Cmalw„ + awhawb(h – KJ

where awh is the lift-curve-slope of the wing-body-nacelle combination.

The aerodynamic forces on any lifting surface can be represented as a lift and drag acting at the mean aerodynamic center, together with a pitching couple independent of the angle of attack (Fig. 2.8). The pitching moment of this force system about the CG is given by (Fig. 2.9)[6]

Mw = MaCw + (Lw cos aw + Dw sin aj(h – hnJc

+ (Lw sin aw – Dw cos ajz (2.2,1)

We assume that the angle of attack is sufficiently small to justify the approximations

cos aw = 1, sin aw = aw

and the equation is made nondimensional by dividing through by pV2Sc. It then be­comes

C,„ = Cmacw + (CLn + C, KaJ(h – hnJ + (CLwaw – CDJzJc (2.2,2)

Although it may occasionally be necessary to retain all the terms in (2.2,2), experi­ence has shown that the last term is frequently negligible, and that CDwaw may be ne­glected in comparison with CLu. With these simplifications, we obtain

Cm„ ~ Cmacw + CLw(h – hnJ

= Стаск + “»aw(h ~ KJ (2.2,3)

where a„ = CLa is the lift-curve-slope of the wing.

Equation 2.2,3 will be used to represent the wing pitching moment in the discus­sions that follow.

The total lift and pitching moment of an airplane are, in general, functions of angle of attack, control-surface angle(s), Mach number, Reynolds number, thrust coefficient, and dynamic pressure.[5] (The last-named quantity enters because of aeroelastic ef­fects. Changes in the dynamic pressure (pV2), when all the other parameters are con­stant, may induce enough distortion of the structure to alter Cm significantly.) An ac­curate determination of the lift and pitching moment is one of the major tasks in a static stability analysis. Extensive use is made of wind-tunnel tests, supplemented by aerodynamic and aeroelastic analyses.

For purposes of estimation, the total lift and pitching moment may be synthe­sized from the contributions of the various parts of the airplane, that is, wing, body, nacelles, propulsive system, and tail, and their mutual interferences. Some data for estimating the various aerodynamic parameters involved are contained in Appendix B, while the general formulation of the equations, in terms of these parameters, fol­lows here. In this chapter aeroelastic effects are not included. Hence the analysis ap­plies to a rigid airplane.

The possible solutions for a suitable configuration are readily discussed in terms of the requirements on Cmo and dCJda. We state here without proof (this is given in Sec. 2.3) that dCJda can be made negative for virtually any combination of lifting surfaces and bodies by placing the center of gravity far enough forward. Thus it is not the stiffness requirement, taken by itself, that restricts the possible configurations, but rather the requirement that the airplane must be simultaneously balanced and have positive pitch stiffness. Since a proper choice of the CG location can ensure a nega­tive dCJda, then any configuration with a positive Cmo can satisfy the (limited) con­ditions for balanced and stable flight.

Figure 2.5 shows the Cmo of conventional airfoil sections. If an airplane were to consist of a straight wing alone (flying wing), then the wing camber would determine the airplane characteristics as follows:

Negative camber—flight possible at a > 0; i. e., CL> 0 (Fig. 2.3a).

Zero camber—flight possible only at a — 0, or CL = 0.

Positive camber—flight not possible at any positive a or CL.

For straight-winged tailless airplanes, only the negative camber satisfies the con­ditions for stable, balanced flight. Effectively the same result is attained if a flap, de­flected upward, is incorporated at the trailing edge of a symmetrical airfoil. A con­ventional low-speed airplane, with essentially straight wings and positive camber, could fly upside down without a tail, provided the CG were far enough forward (ahead of the wing mean aerodynamic center). Flying wing airplanes based on a straight wing with negative camber are not in general use for three main reasons:

1. The dynamic characteristics tend to be unsatisfactory.

2. The permissible CG range is too small.

3. The drag and CLmm characteristics are not good.

The positively cambered straight wing can be used only in conjunction with an auxiliary device that provides the positive Cmo. The solution adopted by experi­menters as far back as Samuel Henson (1842) and John Stringfellow (1848) was to add a tail behind the wing. The Wright brothers (1903) used a tail ahead of the wing (canard configuration). Either of these alternatives can supply a positive Cmu, as illus­trated in Fig. 2.6. When the wing is at zero lift, the auxiliary surface must provide a nose-up moment. The conventional tail must therefore be at a negative angle of at­tack, and the canard tail at a positive angle.

An alternative to the wing-tail combination is the swept-back wing with twisted tips (Fig. 2.7). When the net lift is zero, the forward part of the wing has positive lift, and the rear part negative. The result is a positive couple, as desired.

A variant of the swept-back wing is the delta wing. The positive Cmo can be achieved with such planforms by twisting the tips, by employing negative camber, or by incorporating an upturned tailing edge flap.

Suppose that the airplane of curve a on Fig. 2.3 is disturbed from its equilibrium attitude, the angle of attack being increased to that at В while its speed remains unal­tered. It is now subject to a negative, or nose-down, moment, whose magnitude corre­sponds to BC. This moment tends to reduce the angle of attack to its equilibrium value, and hence is a restoring moment. In this case, the airplane has positive pitch stiffness, obviously a desirable characteristic.

On the other hand, if Cm were given by the curve b, the moment acting when dis­turbed would be positive, or nose-up, and would tend to rotate the airplane still far­ther from its equilibrium attitude. We see that the pitch stiffness is determined by the sign and magnitude of the slope dCJda. If the pitch stiffness is to be positive at the equilibrium a, Cm must be zero, and dCJda must be negative. It will be appreciated from Fig. 2.3 that an alternative statement is “Cmo must be positive, and dCm/da neg­ative if the airplane is to meet this (limited) condition for stable equilibrium.” The various possibilities corresponding to the possible signs of Cmo and dCJda are shown in Figs. 2.3 and 2.4.

An airplane can continue in steady unaccelerated flight only when the resultant external force and moment about the CG both vanish. In particular, this requires that the pitching moment be zero. This is the condition of longitudinal balance. If the pitching moment were not zero, the airplane would experience a rotational accelera­tion component in the direction of the unbalanced moment. Figure 2.3 shows a typi­cal graph of the pitching-moment coefficient about the CG1 versus the angle of attack for an airplane with a fixed elevator (curve a). The angle of attack is measured from the zero-lift line of the airplane. The graph is a straight line except near the stall. Since zero Cm is required for balance, the airplane can fly only at the angle of attack marked A, for the given elevator angle.

‘Unless otherwise specified, Cm always refers to moment about the CG.

The basic flight condition for most vehicles is symmetric steady flight. In this condi­tion the velocity and force vectors are as illustrated in Fig. 2.1. All the nonzero forces and motion variables are members of the set defined as “longitudinal.” The two main aerodynamic parameters of this condition are V and a.

Nothing can be said in general about the way the thrust vector varies with V and a, since it is so dependent on the type of propulsion unit—rockets, jet, propeller, or turboprop. Two particular idealizations are of interest, however,

1. T independent of V, that is, constant thrust; an approximation for rockets and pure jets.

2. 7У independent of V, that is, constant power; an approximation for reciprocat­ing engines with constant-speed propellers.

The variation of steady-state lift and drag with a for subsonic and supersonic Mach numbers (M < about 5) are characteristically as shown in Fig. 2.2 for the range of attached flow over the surfaces of the vehicle (McCormick, 1994; Miele, 1962; Schlichting and Truckenbrodt, 1979). Over a useful range of a (below the stall) the coefficients are given accurately enough by

(2-1,1)

(2.1,2)

The three constants CLn, CDmjn, К are principally functions of the configuration shape, thrust coefficient, and Mach number.

Significant departure from the above idealizations may, of course, be anticipated in some cases. The minimum of CD may occur at a value of a > 0, and the curvature of the C[ vs. a relation may be an important consideration for flight at high CL. When the vehicle is a “slender body,” for example, a slender delta, or a slim wingless

W

Figure 2.1 Steady symmetric flight.

body, the CL curve may have a characteristic upward curvature even at small a (Flax and Lawrence, 1951). The upward curvature of CL at small a is inherently present at hypersonic Mach numbers (Truitt, 1959). For the nonlinear cases, a suitable formula­tion for CL is (USAF, 1978)

CL = (ІСдг. sin 2a + CNaa sin a jsin aj) cos a (2.1,3)

where CNa and CNaa are coefficients (independent of a) that depend on the Mach number and configuration. [Actually CN here is the coefficient of the aerodynamic force component normal to the wing chord, and CNa is the value of CLa at a = 0, as can easily be seen by linearizing (2.1,3) with respect to a.] Equation (2.1,2) for the drag coefficient can serve quite well for flight dynamics applications up to hyper­sonic speeds (M > 5) at which theory indicates that the exponent of CL decreases from 2 to f. Miele (1962) presents in Chap. 6 a very useful and instructive set of typical lift and drag data for a wide range of vehicle types, from subsonic to hyper­sonic.

Part 1

2.1 General Remarks

A general treatment of the stability and control of airplanes requires a study of the dynamics of flight, and this approach is taken in later chapters. Much useful informa­tion can be obtained, however, from a more limited view, in which we consider not the motion of the airplane, but only its equilibrium states. This is the approach in what is commonly known as static stability and control analysis.

The unsteady motions of an airplane can frequently be separated for convenience into two parts. One of these consists of the longitudinal or symmetric motions; that is, those in which the wings remain level, and in which the center of gravity moves in a vertical plane. The other consists of the lateral or asymmetric motions; that is, rolling, yawing, and sideslipping, while the angle of attack, the speed, and the angle of elevation of the x axis remains constant.

This separation can be made for both dynamic and static analyses. However, the results of greatest importance for static stability are those associated with the longitu­dinal analysis. Thus the principal subject matter of this and the following chapter is static longitudinal stability and control. A brief discussion of the static aspects of di­rectional and rolling motions is contained in Secs. 3.9 and 3.11.

We shall be concerned with two aspects of the equilibrium state. Under the head­ing stability we shall consider the pitching moment that acts on the airplane when its angle of attack is changed from the equilibrium value, as by a vertical gust. We focus our attention on whether or not this moment acts in such a sense as to restore the air­plane to its original angle of attack. Under the heading control we discuss the use of a longitudinal control (elevator) to change the equilibrium value of the angle of attack.

The restriction to angle of attack disturbances when dealing with stability must be noted, since the applicability of the results is thereby limited. When the aerody­namic characteristics of an airplane change with speed, owing to compressibility ef­fects, structural distortion, or the influence of the propulsive system, then the airplane may be unstable with respect to disturbances in speed. Such instability is not pre­dicted by a consideration of angle of attack disturbances only. (See Fig. 1.3d, and identify speed with x, angle of attack with y.) A more general point of view than that adopted in this chapter is required to assess that aspect of airplane stability. Such a viewpoint is taken in Chap. 6. To distinguish between true general static stability and the more limited version represented by Cm vs. a, we use the term pitch stiffness for the latter.

Although the major portion of this and the following chapter treats a rigid air­plane, an introduction to the effects of airframe distortion is contained in Sec. 3.5.