Delay time
Delay time is simply defined as the time between an engine failure and the pilot commencing corrective action by rapidly lowering the collective lever. Hence the maximum delay time is the delay that causes the rotor speed to reach the minimum power-off transient value before rising to achieve a stable autorotative condition. Since entry into power-off flight will inevitably involve some change in rotor speed it is common practice to relate key instances to the corresponding rotor speed, see Fig. 2.26.
Having worked-up to a repeatable collective lever lowering rate (by routinely acquiring zero ‘g’ for example) and with reference to a sensitive rotor speed gauge a test pilot will incrementally reduce NRl until NR3 equates to the transient power-off minimum rotor speed. Alternatively the test may be curtailed before the minimum is reached if a sufficiently long delay time has been demonstrated. The model developed in the previous section can be used to show the effect of increasing delay time, see Fig. 2.27. Note that a maximum delay of 1.5 s is possible with the example rotor. Figure 2.28 shows the rotor speeds NRl and NRj in the form of an in-flight cross-plot or ‘how – goes-it ’ chart. Test teams often use this style of chart to get some idea of the effect of increasing delay time on the minimum rotor speed. They can, therefore, ensure that the minimum rotor speed limit is approached in a safe manner by using the chart to select the next incremental reduction in NR . It is worth reminding the reader that in flight the test team will use values of NR to progress the evaluation. The delay times would be determined from appropriate post-flight analysis.











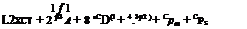
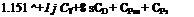 PMR — pAMR ()R)Mr
PMR — pAMR ()R)Mr