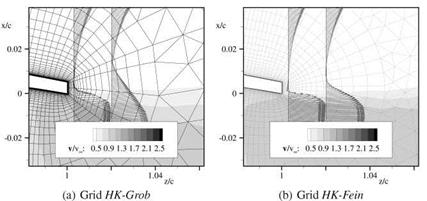
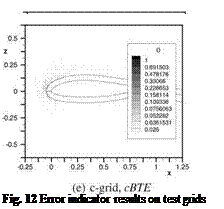
Application of Hexfine and Results
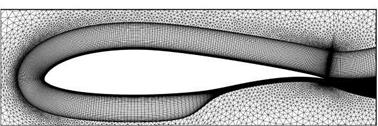
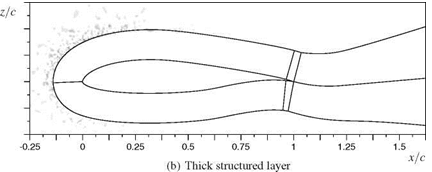
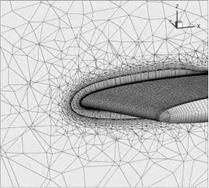
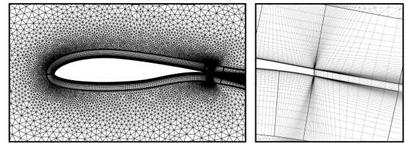
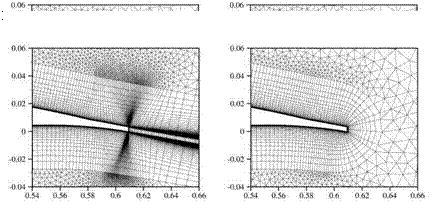
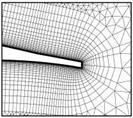
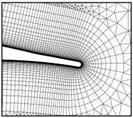
To test the method of local hexahedra refinement a set of two main grids were considered. The first one is the grid cBTE-v2 (including its coarse and fine derivatives) shown in Figure 21(a) which is a c-type grid and has 37,871 grid points. The second grid oBTE shown in Figure 21(b) was derived from cBTE-v2 (including a finer derivative) by remeshing the region around the trailing edge and the wake in o-type. At the trailing edge a structured block was attached to obtain a structured domain reaching far into the wake. It has a total number of 30,127 grid points.
 |
 |
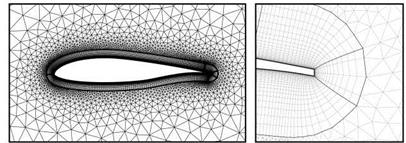
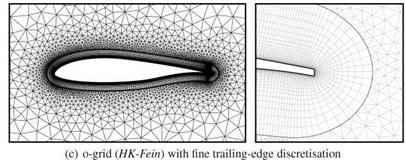
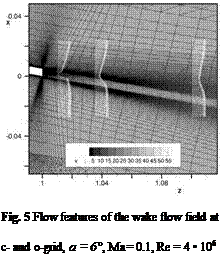
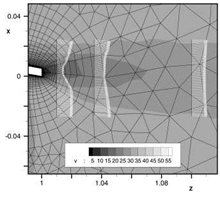
During the first run, the structured wake block of oBTE was refined twice. The result of the refinement is shown in Figure 21(c) in comparison to the initial grid in Figure 21(b). This local refinement had the aim to increase the number of grid points in the wake in order to better capture and preserve the flow field gradients. The quadrilaterals at the trailing-edge surface were not affected by the refinement (cf. Figure 22(a) and 22(b)). The number of grid points was raised by about 28% to 38,680 grid points. In a second run, oBTE was refined three times. This time, the
(c) Topology of the o-grid after refined (d) Topology of the o-grid after refined twice thrice
 |
 |
 |
Fig. 22 Comparison of the grid topology at the trailing edge
 |
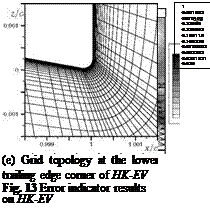
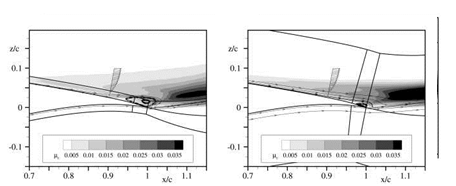
first refinement affected quadrilaterals up to the wall (cf. Figure 22(c)). The second and third refinement affected a portion of the grid downstream of the trailing edge. The resulting wake grid topology is shown in Figure 21(d). In addition, the grid was refined another three times around the upper corner of the trailing edge with the intention to better discretise the sharp corner. Flow solutions were obtained on
 |
 |
 |
Fig. 24 Grid topology and flow condition at the upper trailing edge corner
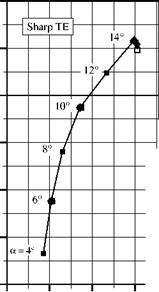
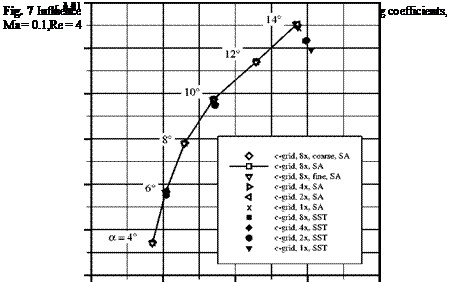
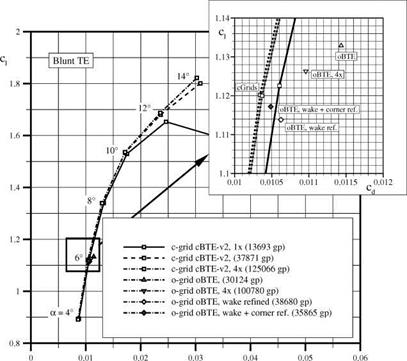
these grids. The resulting polar curves are shown in Figure 23. For a = 6°, the grid cBTE-v2, which is regarded as the reference grid, yields a grid converged solution since the differences in lift and drag to the finer grid are negligible. The coefficients of oBTE differ by 1.30 lift counts and 10.90 drag counts to the reference grid.
The o-grid with the refined wake reduces the differences to 0.60 lift counts and 2.80 drag counts. This is an improvement of cd by almost 75%. Analyses showed that refining the wake region a fourth or a fifth time does not yield any appreciable improvements. This reveals that the errors in the flow solution due to a o-topology cannot be attributed to a poor wake discretisation alone. In fact, the coarse discretisation of sharp corners with o-grids constitutes a further source of error. Analysing the flow features very close to the trailing edge in Figure 24(a) and 24(b) edge reveals, that the way the o-grid discretises the trailing edge is not sufficient to capture the sharp corner. This leads to very different flow features at the trailing edge. Figure 24(c) shows that the o-grid with refined wake and the additional corner refinement can capture the sharp corner much better, thus leading to less differences in the predicted flow features. As a result, the o-grid with the additionally refined upper corner has a difference of only 0.30 lift counts and 1.40 drag counts (cf. polar curve in Figure 23). To sum it up, by well-directed local refinement the error in c could be reduced from 1.30 to 0.3 lift counts whereas cd could be reduced from 10.90 drag counts to 1.40 drag counts. This is achived by increasing the number of grid points at about only 19%. In contrast, not even a unified grid refinement by doubling the number of grid points in both coordinate directions, which results in a large increase of the total number of grid points by 345.0%, does give a comparable improvement.