We assume the flow cases under consideration to be in the continuum regime, and at most at its border, in the slip-flow regime, Section 2.3. Nevertheless, we used in our above discussion the concept “excitation by collisions”. We apply this concept now in order to get a basic understanding of rate processes, i. e., excitation or reaction processes, which in principle are time dependent.
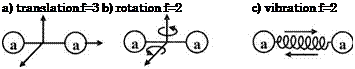
The number of collisions per unit time depends on the density and the temperature of the gas. The number of collisions at which molecules react is much less than the number of collisions they undergo, because only a fraction of the collisions involves sufficient energy (activation factor), and only a fraction of collisions with sufficient energy actually leads to a reaction (steric factor) [1]. The orders of magnitude of the number of collisions needed to reach equilibrium of degrees of freedom or to dissociate molecules [1] are given in the following Table 5.2.
|
Table 5.2. Number of collisions to reach equilibrium of degrees of freedom or dissociation.
|
Phenomenon
|
Number of collisions
|
|
Translation
|
0(10)
|
|
Rotation
|
0(10)
|
|
Vibration
|
O(104)
|
|
Dissociation
|
> O(104)
|
|
The table shows that only a few collisions are needed to obtain full excitation of translation and rotation.[47] Knowing the very low characteristic rotational temperatures of the diatomic species N2, O2, NO, Appendix B.1, we understand why for our flow cases in general rotational degrees of freedom can be considered as fully excited. Vibrational excitation needs significantly more collisions, and dissociation still more. We note that this consideration is valid also for the reverse processes, i. e., de-excitation of internal degrees of freedom, and recombination of atomic species.
Since excitation is a process in time, we speak about the “characteristic excitation or reaction time” т, which is needed to have the necessary collisions to excite degrees of freedom or to induce reactions. Comparing this time with a characteristic flow time leads us to the consideration of thermal and chemical rate processes.
We introduce the first Damkohler number DAM 1 [8]:
DAMl = —. (5.22)
T
Here tres is the residence time of a fluid particle with its atoms and molecules in the considered flow region, which we met in another context already in Section 4.1
tres = —• (5.23)
vref
Lref is a characteristic length of the flow region under consideration, and vref a reference speed, Fig. 4.2.
The characteristic time
t = t(p, T, initial state) (5.24)
is the excitation time of a degree of freedom or the reaction time of a species, and depends on the density, the temperature and the initial state regarding the excitation or reaction [1].
We distinguish the following limiting cases:
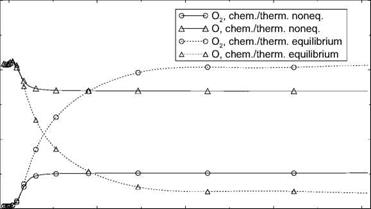
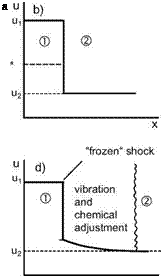
— DAM 1 ^ to: the residence time is much larger than the characteristic time: tres ^ t, the considered thermo-chemical process is in “equilibrium”, which means that each of the internal degrees of freedom and the chemical reactions is in equilibrium. Then the actual vibrational energy or the mass fraction of a species is a function of local density and temperature only: evibri = evibri(p, T), ші = ші(р, T). In this case we speak about “equilibrium flow” or “equilibrium real gas”.
— DAM 1 ^ 0: the residence time is much shorter than the characteristic time: tres ^ t, the considered thermo-chemical process is “frozen”. In the flow region under consideration practically no changes of the excitation state or the mass fraction of a species happen: e. g., evibri = evibri, ші = шіхі. We speak about “frozen flow” or “frozen real gas”. In Sub-Section
5.5.1 an example is discussed.
— DAM 1 = 0(1): the residence time is of the order of the characteristic time, the considered process is in “non-equilibrium”. In the flow region under consideration the thermo-chemical processes lag behind the local flow changes, i. e., the process is a relaxation process. We call this “nonequilibrium flow” or “non-equilibrium real gas”. We discuss an example in Sub-Section 5.5.
Of course for the aerothermodynamic problems at hand it is an important question whether the thermo-chemical rate process is relevant energetically. The parameter telling this is the second Damkohler number DAM2:
which compares the energy involved in the non-equilibrium process qne with the total energy of the flow H0. If DAM2 ^ 0, the respective rate process in general can be neglected.
We note that the Damkohler numbers must be applied, like any similarity parameter, with caution. A global Damkohler number DAM 1 ^ ж can indicate, that the flow past a flight vehicle globally is in equilibrium. However, locally a non-equilibrium region can be embedded. This can happen, for example, behind the bow shock in the nose region of a flight vehicle, but also in regions with strong flow expansion. At a RV at large angle of attack, for instance, we have the situation that the gas in the stagnation point region is heated and is in some thermo-chemical equilibrium or non-equilibrium state. A part of this gas will enter the windward side of the flight vehicle, where only a rather weak expansion happens. Hence we will have equilibrium or non-equilibrium flow effects also here. The part entering the leeward side on the other hand undergoes a strong expansion. Hence it is to be expected that frozen flow exists there, with a frozen equilibrium or non-equilibrium state more or less similar to that in the stagnation region.
We note finally that in the flow of a gas mixture the Damkohler numbers of each of the thermo-chemical rate processes must be considered separately, since they can be vastly different. This would imply that a multi-temperature model, with, for instance, the translational temperature Ttrans, and vibrational temperatures Tvibri(i = N2,O2,NO), and appropriate transport equations, Appendix A, must be employed, see, e. g., [7]. As a consequence of multi-temperature models one has to be careful in defining the local speed of sound of a flow, which is important for the stability of discrete computation methods, but also for the presentation of experimental data with the Mach number as parameter [1, 7].
In the following we consider shortly the basics of rate processes of vibrational excitation, and chemical reactions. For a thorough presentation see,
e. g., [1, 7].
For the vibrational rate process of a diatomic species we can write in general [1]
d’evibr _ evibr evibr fr
dt t * ’
Here evibr is the actual vibrational energy, e*vibr the equilibrium energy, and t the relaxation time. The equilibrium energy e*vibr is a function of the temperature T, eq. (5.13). The relaxation time t is a function of temperature and pressure/density, as well as of characteristic data ki, k2, Ovibr of the species (Landau-Teller theory [1]):
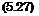 ki T 5/6e(k2/T )1/3
ki T 5/6e(k2/T )1/3
p(1 — Є Є^іЬт/T ) ’
which for sufficiently low temperatures reads
e(k2/T )1/3
t = c—————— . (5-28)
P
Eq. (5.26) shows, since т > 0, that the vibrational energy always tends towards its equilibrium value. The relation in principle holds for large nonequilibrium, but due to the underlying assumption of an harmonic oscillator, it actually is limited to small, not accurately defined, departures from equilibrium [1].
Above it was said that full vibrational excitation cannot be reached, because the molecule will dissociate before this state is reached. The transition to dissociation, i. e., vibration-dissociation coupling, can be modeled to different degrees of complexity, see, e. g., [9]—[11]. Often it is neglected in computation methods, because it is not clear how important it is for the determination of flow fields, forces, thermal loads, etc.
Chemical rate processes without vibration-dissociation coupling can be described in the following way. For convenience we consider only the so called thermal dissociation/recombination of nitrogen [7]
N2 + M ^ 2N + M. (5.29)
Here M is an arbitrary other gas constituent. For the dissociation reaction (forward reaction: arrow to the right) it is the collision partner, which lifts the energy level of N2 above its activation, i. e., dissociation, level. For the recombination reaction (backward reaction: arrow to the left) it is the already mentioned “third body” M, which carries away the dissociation energy and conserves also the momentum balance.
The rate of change equation for an
This equation sums up all individual reactions r, which add to the overall rate of change of the species, i. e., the contributions of the reactions with different third bodies M. In the case of the thermal dissociation/recombination of nitrogen, eq. (5.29), we have five reactions (r = 5), with M being N2, N, O2, O, NO.
In eq. (5.30) v’ir and v"r are the stoichiometric coefficients of the forward () and the backward (") reactions r of the species i with the third body M. The forward reaction rate of reaction r is kfr, and the backward rate kbr.
If a reaction r is in equilibrium, dcir/dt ^ 0, eq. (5.30) yields
kfr ГГ C – " = kfer ГГ ci " , (5.31)
ii
|
and the equilibrium constant
|
к – ^
c hr ■
|
(5.32)
|
|
The equilibrium constant of each reaction can be approximated by the modified Arrhenius equation [1]
|
|
Kc = CcTnc e-Gdiss/T,
|
(5.33)
|
|
with Odiss being the characteristic dissociation temperature. reaction rate is approximated by
|
The forward
|
|
kfr = Cf Tnf е-Єлі^^/т,
|
(5.34)
|
|
so that the backward reaction rate is
|
|
|
hr =
Cc
|
(5.35)
|
|
In these equations Cc, Cf, цс, Vf are independent of the temperature T
[1]-
We note that (global) equilibrium flow is only given, if all DamkOhler numbers DAM 1i ^ 0, which means that all reactions r of all species i are in equilibrium.
The constants and characteristic data for the above relations can be found in [1, 7]. Regarding a critical review of the state of the art see, e. g., [9].











 0.15
0.15
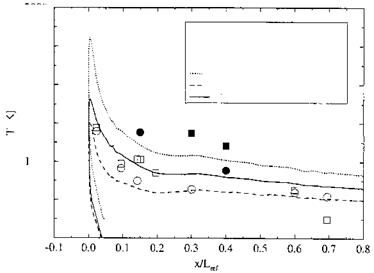
![Surface Catalytic Recombination Подпись: Table 5.8. Parameters of the (laminar) flow past the X-38 at 60 km altitude, and surface material (S: downward deflection angle of the body flap) [20]. Moo Vco [m/s] ЯЄСО }L L [m] a [°] 4 П Є Nose cone Body Body flap 20 6,085.5 6.2-Ю5 9.14 40 20 0.87 SiC SiOn SiC](/img/3130/image303_4.gif)

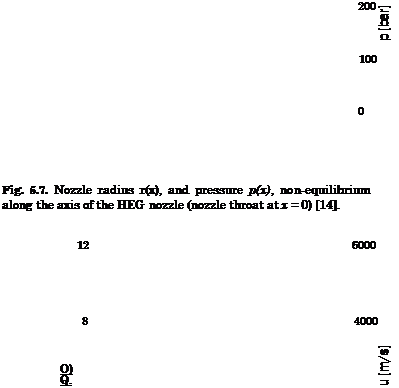
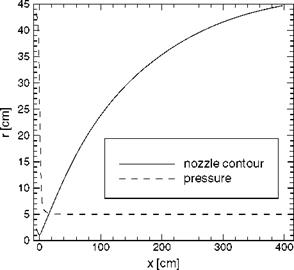

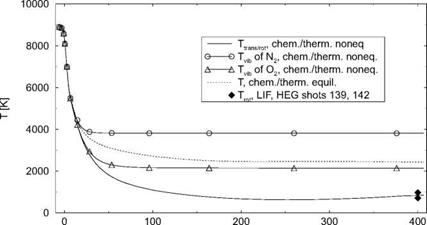
 0
0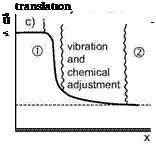
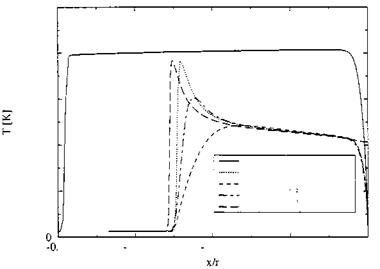 (we will come back to this phenomenon in Sub-Section 6.4.1). The temperature behind the shock is nearly constant. It reaches shortly ahead of the cylinder approximately the total temperature T0 = 8,134 K and drops then in the thermal boundary layer to the prescribed wall temperature Tw = 600 K.[48]
(we will come back to this phenomenon in Sub-Section 6.4.1). The temperature behind the shock is nearly constant. It reaches shortly ahead of the cylinder approximately the total temperature T0 = 8,134 K and drops then in the thermal boundary layer to the prescribed wall temperature Tw = 600 K.[48]