Category MECHANICS. OF FLIGHT

As stated above, the pitot and static tube combination provides a means of measuring the dynamic pressure. It does not tell us the speed directly, but we can work out the speed if we know the density. Until recently, there was no simple method of measuring density, so all that could be done was to use the dynamic pressure-measuring device described above, and mark on the dial the speed that this dynamic pressure would correspond to at standard sea-level air density. This instrument is called the Air Speed Indicator (ASI). You will see that since the instrument is calibrated assuming the standard sea-level value of air density, it does not give the true speed, unless the aircraft is flying at a height where the density just happens to be equal to the standard sea-level value.

Nowadays, there are devices which can measure the true air speed, but the air speed indicator described above is still an important item on any instru­ment panel. This is because the lift and other forces on the aircraft are dependent on dynamic pressure, and the air speed indicator gives a reading which is directly related to dynamic pressure. For example, if the dynamic pressure is too small, the wings will not be able to generate enough lift to keep the aircraft in steady level flight. The value of the dynamic pressure and hence the indicated speed at which this occurs will always be the same whatever the height. The pilot just has to remember to keep above this minimum indicated speed. If the pilot had only a true speed indicator, he would have to know what the minimum speed was at every height.

If instead of connecting the static pressure vent and the pitot tube to two sep­arate pressure-measuring devices, we connect them across one device which measures the difference in pressure then, from the above expression, we can see that we will obtain a measurement of the dynamic pressure. Thus we have a simple means of measuring the dynamic pressure, and if only we could find a way of measuring or assessing the density, we could determine the flow speed, but more of this later; let us first concentrate on the measurement of dynamic pressure, which we will show is actually just as important to the pilot as the speed.

The pressure difference measuring device used on aircraft consists of either a diaphragm or a capsule (similar to the type used in an aneroid barometer). The stagnation pressure is applied to one side, and the static pressure is applied to the other. The resulting deflection of the diaphragm can then either be amplified through a series of levers to cause a dial pointer to move, as on older mechanical devices, or can be used to produce a proportional electrical output to be fed into an appropriate electronic circuit. This instrument thus gives a reading that is proportional to the dynamic pressure, but as we shall see, it forms the basis of the air speed indicator.

The pitot tube and static pressure hole are located at a suitable convenient position on the aircraft. The location of the static tapping is very important because it is essential to choose a position where the local static pressure is about the same as that in the free stream away from the aircraft. We need, therefore, to find a place where the flow speed is about the same as that in the free stream, and also is not too sensitive to change in the direction that the air­craft is pointing. The pitot and static holes are normally heated to avoid icing at low temperatures.

As an alternative to using separate pitot and static tubes, it is possible to use a combined device called the pitot-static tube which is illustrated in Fig. 2.5. The pitot-static tube consists of two concentric tubes. The inner one is simply a pitot tube, but the outer one is sealed at the front and has small holes in the

Static

tube

Fig 2.5 Concentric pitot-static tube

side to sense the static pressure. The pitot-static tube is a very convenient device, and by mounting it on the wingtips or the nose it can be arranged so that it is well clear of interference from the flow around the aircraft. Pitot – static tubes are always used for accurate speed measurement on prototypes, but for civil and private aircraft separate pitot and static tappings are normally used. Pitot-static tubes are frequently used in wind tunnels.

Bernoulli’s equation gives rise to a simple method of measuring air speed. You can see that the dynamic pressure is related to the density and the speed, (dynamic pressure = ypV2), so if we could measure the dynamic pressure and the density we could determine the speed. Fortunately there is a very simple way of measuring the dynamic pressure at least.

If we point a tube directly into the flow of air, and connect the other end to a pressure-measuring device, then that device will read the stagnation pressure. The reason for this is that the tube is full of air and its exit is blocked, so no air can flow down the tube; the oncoming air therefore is brought to rest rela­tive to the tube as it meets the open end of the tube. This type of tube is called a pitot tube and provides a means of measuring stagnation pressure. A dif­ferent result is obtained if we make a hole in the side of a wind tunnel or in the fuselage of an aircraft, and connect this via a tube to a pressure-measuring device. The hole will not impede the flow of air, so the pressure measured will be the local static pressure. A hole used for this purpose is called a static vent or tapping. Since static pressure plus dynamic pressure equals stagnation pressure as shown above, it follows that

An aircraft flying through the air causes local changes in both velocity relative to the aircraft and pressure. These changes are linked by Bernoulli’s equation. This equation can be written in many forms, but originally it was given by

t) V2

t – + — + gz = a constant

P 2

where z is the height, p is the pressure, p is the density, У is the flow speed, and g is the gravity constant.

The equation is sometimes called Bernoulli’s integral, because it is obtained by integrating the Euler momentum equation for the case of a fluid with con­stant density. Since the equation involves a constant density, it should really only be applied to incompressible fluids. Completely incompressible fluids do not actually exist, although liquids are very difficult to compress. Air is defi­nitely compressible, but nevertheless, airflow calculations using Bernoulli’s equation give good answers unless the speed of the flow starts going above about half the speed of sound. Bernoulli’s equation will also not apply in regions where viscosity is important.

The terms in this equation all have the units of energy per unit mass, and the equation looks temptingly similar to the steady flow energy equation that you will meet if you ever study thermodynamics. The second and third terms do in fact represent kinetic energy per unit mass and potential energy per unit mass respectively. The true energy equation is, however, significantly different, and contains an important extra term, internal energy, which cannot be neg­lected in compressible airflows. However, whatever Bernoulli’s equation is or is not, it remains a useful and simple means for getting approximately correct answers for low-speed flows. Aerodynamicists usually prefer it in the form below:

p + IpV2 + pgz = a constant

This is obtained by multiplying the original equation by the density, which makes all of the terms come out in the units of pressure (N/m2). The last term is usually ignored because changes in height are small in most of the calcula­tions that we perform for airflows around an aircraft, so we write

p + IpV2 = a constant

The first term represents the local pressure of the air, and is called the static pressure. The second term jpV2 is associated with the flow speed and is called dynamic pressure. For convenience it is sometimes represented by the letter q, but in this book we will use the full expression.

If we ignore the third term, as above, Bernoulli’s equation says that adding the first two terms, the static and the dynamic pressure, produces a constant result. Therefore, if the flow is slowed down so that the dynamic pressure decreases, then to keep the equation in balance the static pressure must increase. If we bring the flow to rest at some point, then the pressure must reach its highest possible value, because the dynamic pressure becomes zero. This maximum value is called the stagnation pressure because it occurs at a point where the air has stopped or become stagnant. Using the version of Bernoulli’s equation above, we can write

p + IpV2 = a constant = p (stagnation) + 0 This gives the important result that

Static pressure + Dynamic pressure = Stagnation pressure

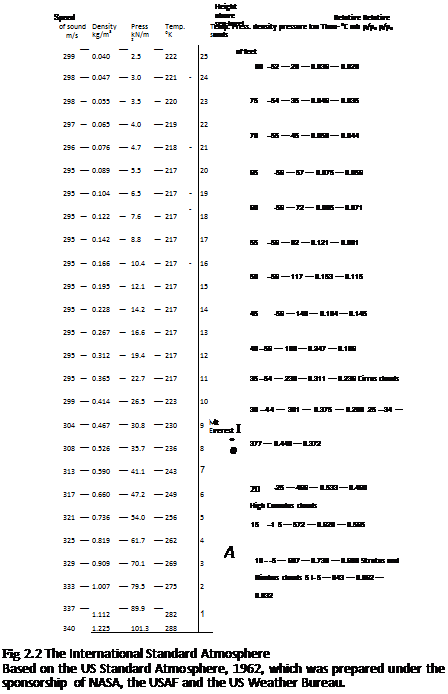
The reader will have realised that there is liable to be considerable variation in those properties of the atmosphere with which we are concerned – namely, temperature, pressure and density. Since the performance of engine, aeroplane and propeller is dependent on these three factors, it will be obvious that the actual performance of an aeroplane does not give a true basis of comparison with other aeroplanes, and for this reason the International Standard Atmosphere has been adopted. The properties assumed for this standard atmosphere in temperate regions are those given in Fig. 2.2, earlier. If, now, the actual performance of an aeroplane is measured under certain conditions of temperature, pressure and density, it is possible to deduce what would have been the performance under the conditions of the Standard Atmosphere, and thus it can be compared with the performance of some other aeroplane which has been similarly reduced to standard conditions.

The altimeter

The instrument normally used for measuring height is the altimeter, which was traditionally merely an aneroid barometer graduated in feet or metres instead of millimetres of mercury or millibars. As a barometer it will record the pressure of the air, and since the pressure is dependent on the temperature as well as the height, it is only possible to graduate the altimeter to read the height if we assume certain definite conditions of temperature. If these con­ditions are not fulfilled in practice, then the altimeter cannot read the correct height. Altimeters were at one time graduated on the assumption that the tem­perature remained the same at all heights. We have already seen that such an assumption is very far from the truth, and the resulting error may be as much as 900 m at 9000 m, the altimeter reading too high owing to the drop in tem­perature. Altimeters are now calibrated on the assumption that the temperature drops in accordance with the International Standard Atmosphere; this method reduces the error considerably, although the reading will still be incorrect where standard conditions do not obtain.

As a barometer the altimeter will be affected by changes in the pressure of the atmosphere, and therefore an adjustment is provided, so that the scale can be set (either to zero or to the height of the airfield above sea-level) before the commencement of a flight, but in spite of this precaution, atmospheric con­ditions may change during the flight, and it is quite possible that on landing on the same airfield the altimeter will read too high if the pressure has dropped in the meantime, or too low if the pressure has risen.

Although it is convenient to use SI units for calculations and numerical examples, it should be remembered that knots and feet are still the inter­nationally approved units for speed and altitude respectively when dealing with aircraft operations. If you ever sit at the controls of an aircraft you will almost certainly find that the altimeter is calibrated in feet. We shall therefore use feet (ft) when referring to this instrument.

Modern altimeters are much more sensitive than the old types; some, instead of having one pointer, may have as many as three, and these are geared together like the hands of a clock so that the longest pointer makes one revol­ution in 1000 ft, the next one in 10 000 ft, and the smallest one in 100 000 ft. Unfortunately this has sometimes proved ‘too much of a good thing,’ and acci­dents have been caused by pilots mistaking one hand for another. The more modern tendency is to show the height level in actual figures in addition to one or more pointers. But, like a sensitive watch, the altimeter is of little use unless it can be made free from error, and can be read correctly. In the modern types, if the pilot sets the altimeter to read zero height, which he can do simply by turning a knob, a small opening on the face of the instrument discloses the pressure of the air at that height – in other words, the reading of the barom­eter. Conversely – and this is the important point – if, while in the air, he finds out by radio the barometric pressure at the airfield at which he wishes to land, he can adjust the instrument so that this pressure shows in the small opening, and he can then be sure that his altimeter is reading the correct height above that aerodrome, and that when he ‘touches down’ it will read zero. The altimeter may be used in this way for instrument flying and night flying, that is to say when the height above the ground in the vicinity of the aerodrome is of vital importance, but for ordinary cross-country flying during the day it may be preferable to set the sea-level atmospheric pressure in the opening. Then the pilot will always know his height above sea-level and can compare this with the height, as shown on the map, of the ground over which he is flying. If this method is used, instead of the altimeter reading zero on landing, it will give the height of the aerodrome above sea-level. There is, however, a snag in this method in that the sea-level atmospheric pressure varies from place to place and so different pilots may set their altimeters differently, thereby increasing the risk of collision; for this reason modern practice for flying above 3000 ft is to set the altimeter to standard sea-level pressure of 1013.2 mb, which means, in effect, that all the altimeters may be reading the incorrect height, but that only aircraft flying at the same height can have the same altimeter readings. Above 3000 ft heights are referred to in terms of flight levels (or hundreds of feet), e. g. FL 35 is 3500 ft, FL 40 is 4000 ft, then FL 45, 50, 55, and so on. Increases in flight levels are in fives because of the quadrantal system (in operation in the UK) which determines the height at which the pilot must fly for specific compass headings.

The question of altimeter setting has long been a matter for controversy among pilots – and even among nations.

In recent years there have been radical changes in aircraft instruments and displays. Instead of individual instruments there may now be a computer – screen type of display, but the altimeter display still looks quite similar to the traditional instrument. The information on which the display is based may also still be the external pressure, but there are now alternative, more accurate, height-reading devices such as radio or radar altimeters.

The reader who is particularly interested in altimeters and other instru­ments is referred to Aircraft Instruments by E. H. J. Pallett, a companion volume in the Introduction to Aeronautical Engineering Series.

We have, up to the present, only considered the physical properties of the atmosphere, and, in fact, we are hardly concerned with its chemical or other properties. Air, however, is a mixture of gases, chiefly nitrogen and oxygen, in the proportion of approximately four-fifths nitrogen to one-fifth oxygen. Of the two main gases, nitrogen is an inert gas, but oxygen is necessary for human life and also for the proper combustion of the fuel used in the engine, there­fore when at great heights the air becomes thin it is necessary to provide more oxygen. In the case of the pilot, this was formerly done by supplying him with pure oxygen from a cylinder, but in modern high-flying aircraft, the whole cabin is pressurised so that pilot, crew and passengers can still breathe air of similar density, pressure, temperature and composition as that to which they are accustomed at ground level, or at some reasonable height. As for the engines, it has always been preferable to provide extra air rather than oxygen, because although the oxygen is needed for combustion, the nitrogen provides, as it were, the larger part of the working substance which actually drives the engine. In piston engines the extra air is provided by a process known as super­charging, which means blowing in extra air by means of a fan or fans; in jet aircraft the principle is fundamentally the same, though simpler because the engine is in itself a supercharger and this, combined with the ‘ram effect’ at the higher true speeds achieved at altitude, keeps up the supply of air as necessary.

But our chief concern with the wind at the present moment is that we must understand that when we speak of the speed of an aeroplane we mean its speed relative to the air, or air speed as it is usually termed. Now the existence of a wind simply means that portions of the air are in motion relative to the earth, and although the wind will affect the speed of the aeroplane relative to the earth – i. e. its ground speed – it will not affect its speed relative to the air.

For instance, suppose that an aeroplane is flying from A to В (60 km apart), and that the normal speed of the aeroplane (i. e. its air speed) is 100 km/h (see Fig. 2.3). If there is a wind of 40 km/h blowing from В towards A, the ground speed of the aeroplane as it travels from A to В will be 60 km/h, and it will take one hour to reach B, but the air speed will be 100 km/h. If, when the aero­plane reaches B, it turns and flies back to A, the ground speed on the return journey will be 140 km/h (Fig. 2.4); the time to regain A will be less than half an hour, but the air speed will still remain 100 km/h – that is, the wind will strike the aeroplane at the same speed as on the outward journey. Similarly, if the wind had been blowing across the path, the pilot would have inclined his aeroplane several degrees towards the wind on both journeys so that it would have travelled crabwise, but again, on both outward and homeward journeys the air speed would have been 100 km/h and the wind would have been a headwind straight from the front as far as the aeroplane was concerned.

An aeroplane which encounters a headwind equal to its own air speed will appear to an observer on the ground to stay still, yet its air speed will be high. A free balloon flying in a wind travels over the ground, yet it has no air speed – a flag on the balloon will hang vertically downwards.

All this may appear simple, and it is in fact simple, but it is surprising how long it sometimes takes a student of flight to grasp the full significance of air speed and all that it means. There are still pilots who say that their engine is overheating because they are flying ‘down wind’! It is not only a question of speed, but of direction also; a glider may not lose height in a rising current of air (it may, in fact, gain height), yet it is all the time descending relative to the air. In short, the only true way to watch the motion of an aeroplane is to imagine that one is in a balloon floating freely with the wind and to make all observations relative to the balloon.

Ground speed is, of course, important when the aeroplane is changing from one medium to another, such as in taking-off and landing, and also in the time taken and the course to be steered when flying cross-country – this is the science of navigation, and once again the student who is interested must consult books on that subject.

The reader may have noticed that we have not been altogether consistent, nor true to the SI system, in the units that we have used for speed; these already include m/s, km/h and knots. There are good reasons for this inconsis­tency, the main one being that for a long time to come it is likely to be standard practice to use knots for navigational purposes both by sea and by air, km/h for speeds on land, e. g. of cars, while m/s is not only the proper SI unit but it must be used in certain formulae. We shall continue to use these different units throughout the book as and when each is most appropriate, and the important thing to remember is that it is only a matter of simple conversion from one to the other –

1 knot = 0.514 m/s = 1.85 km/h

The existence of separate regions of high and low pressure is the cause of wind, or bodily movement of large portions of the atmosphere. Winds vary from the extensive trade winds caused by belts of high and low pressure sur­rounding the earth’s surface to the purely local gusts and ‘bumps’, caused by local differences of temperature and pressure. On the earth’s surface we are usually only concerned with the horizontal velocity of winds, but when flying the rising convection currents and the corresponding downward movements of the air are also important. The study of winds, of up-and-down convection currents, of cyclones and anti-cyclones, and the weather changes produced by them – all these form the fascinating science of meteorology, and the reader who is interested is referred to books on that subject.

In the lower regions of the atmosphere conditions are apt to be erratic; this is especially so within the first few hundred feet. It often happens that as we begin to climb the temperature rises instead of falling – called an inversion of temperature. This in itself upsets the stability of the air, and further distur­bances may be caused by the sun heating some parts of the earth’s surface more than others, causing thermal up-currents, and by the wind blowing over uneven ground, hangars, hills, and so on. On the windward side of a large building, or of a hill, the wind is deflected upwards, and on the leeward side it is apt to leave the contour altogether, forming large eddies which may result in a flow of air near the ground back towards the building or up the far side of the hill, that is to say in the opposite direction to that of the main wind. Even when the surface of the ground is comparatively flat, as on the average airfield, the wind is retarded near the ground by the roughness of the surface, and successive layers are held back by the layers below them – due to viscosity
– and so the wind velocity gradually increases from the ground upwards. This phenomenon is called wind gradient. When the wind velocity is high it is very appreciable, and since most of the effect takes place within a few metres of the ground it has to be reckoned with when landing.

Quite apart from this wind gradient very close to the ground, there is often also a wind gradient on a larger scale. Generally, it can be said that on the average day the wind velocity increases with height for many thousands of feet, and it also tends to veer, i. e. to change in a clockwise direction (from north towards east, etc.); at the same time it becomes more steady and there are fewer bumps.

Although air is not quite a ‘perfect gas’ it does obey the gas law within reason­able limits, so we can say that

P = RT P

It is often convenient to express the density in terms of the ratio of the density at some height p to the value at standard sea-level conditions p0. This ratio p/p0 is usually denoted by the Greek letter cr, and is called the relative density.

EXAMPLE 2.1

If the temperature at sea-level in the temperate ISA is 15°C, and the lapse rate is 6.5°C per km, find the temperature and density at 6 km altitude where the pressure is 47 200 N/m2. The gas constant R = 287 J/kg K.

SOLUTION

First we must convert all temperatures to absolute (Kelvin). Sea-level tempera­ture is

273 + 15 = 288 K.

Temperature at 6000 m is 288 + 6.5 X 6 = 249 К The gas law states that

— = RT or p = – Ё-

p K1

so the density at 6 km is

An important property of air in so far as it affects flight is its viscosity. This is a measure of the resistance of one layer of air to movement over the neigh­bouring layer; it is rather similar to the property of friction between solids. It is owing to viscosity that eddies are formed when the air is disturbed by a body passing through it, and these eddies are responsible for many of the phenomena of flight. Viscosity is possessed to a large degree by fluids such as treacle and certain oils, and although the property is much less noticeable in air, it is none the less of considerable importance.

Another change which takes place as we travel upwards through the lower layers of the atmosphere is the gradual drop in temperature, a fact which unhappily disposes of one of the oldest legends about flying – that of Daedalus and his son Icarus, whose wings were attached by wax which melted because he flew too near the sun. In most parts of the world, the atmospheric tempera­ture falls off at a steady rate called the lapse rate of about —6.5°C for every 1000 metres increase in height up to about 11000 metres. Above 11000 metres, the temperature remains nearly constant until the outer regions of the atmosphere are reached. The portion of the atmosphere below the height at which the change occurs is called the troposphere, and the portion above, the stratosphere. The interface between the two is called the tropopause. The lapse rate and the height of the tropopause vary with latitude. In Arctic regions, the rate of temperature change is lower, and the stratosphere does not start until around 15 500 m. The temperature in the stratosphere varies between about — 30°C at the equator to —95°C in the Arctic. In temperate regions such as Europe the temperature in the stratosphere is around —56.5°C.

For aircraft performance calculations, it is normal practice to use a stan­dard set of conditions called the International Standard Atmosphere (ISA). This defines precise values of lapse rate, height of the tropopause, and sea-level values of temperature, pressure and density. For temperate regions the ISA

value of the lapse rate is —6.5°C per 1000 m, the tropopause is at 11 km, and the sea-level values of pressure and temperature are 101.325 kN/m2, and 15°C respectively.

Modern long – and medium-range airliners cruise in or very close to the stratosphere, and the supersonic airliner Concorde used to fly in the strato­sphere well above the tropopause. When piston-engined aircraft first started to fly in the stratosphere, conditions were very uncomfortable for the crew. The low density and pressure meant that oxygen masks had to be worn, and at temperatures of —56°C, even the heavy fur-lined clothing was barely adequate. Nowadays, the cabins of high-flying airliners are pressurised, and the air is heated, so that the passengers are unaware of the external conditions. Nevertheless, above every seat there is an emergency oxygen mask to be used in the event of a sudden failure of the pressurisation system.

Despite the low external air temperature in the stratosphere, supersonic air­craft have the problem that surface friction heats the aircraft up during flight, so means have to be provided to keep the cabin cool enough.