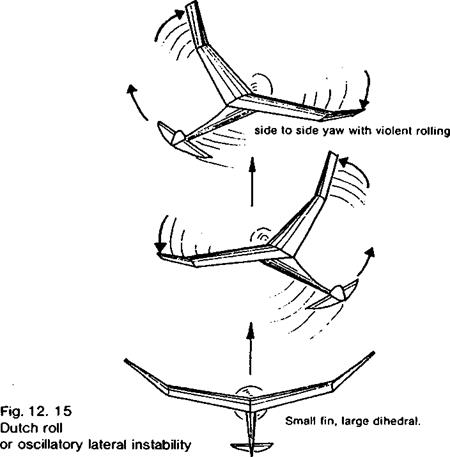
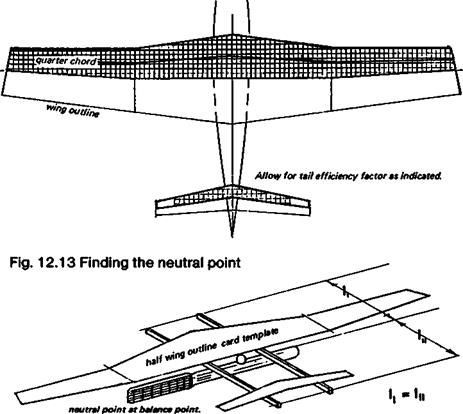
DIHEDRAL
A wing with no dihedral is neutrally stable in roll. Any roll which starts will be damped out, but there will be no tendency to correct the attitude of the model once the roll has been arrested. If one wing is down, it will stay down. Damping in roll has already been mentioned in Chapter 4 (see Fig. 5.9). As the wing rolls, the down-going wing meets the. air at a greater angle of attack while the angle of attack of the up-going wing is reduced by the same amount. There arises an imbalance of lift on the two sides, which tends to bring the roll to a stop. Once the roll does cease, the angle of attack of each wing is the same, so no tendency to return to level flight is present. The model, canted over at an angle,
sideslips.
It is sometimes suggested in the modelling press that dihedral operates to stabilise a model as indicated in Figure 12.17. In level flight the weight, acting vertically down, is supported by the vertical component of lift on the two wings. Since the wings are set at a dihedral angle, the actual lift force has to be resolved as shown, into a relatively large vertical force and a small horizontal component acting inwards. If the model is canted Over by a gust, one wing tends more toward the horizontal position and the other is at a steeper angle. As the diagram shows, the vertical component of the lift on the down side is then larger and that on the higher side, smaller. A corrective restoring moment appears tending to roll the model back to level flight again.
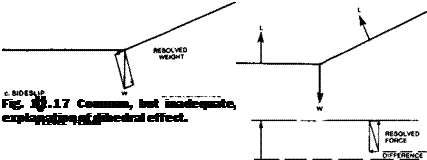 |
Unfortunately, this explanation, although sufficing for small and momentary disturbances, is far from adequate. In a more complete explanation, not only the lift forces but the weight too must be resolved into one component acting at right angles to the axis of the aircraft, the other then slanting toward the down-wing side. This creates an unbalanced situation. As soon as any such banking occurs, the model will begin to sideslip towards the lower wing. The sideslip changes the angles of attack of the two wings differentially and it is this which provides the powerful, corrective rolling force of dihedral.
Figure 12.18 shows how, in a sideslip, the angle of attack of the into-slip wing is increased and that of the other decreased. The lift on the into-slip side increases and a rolling force appears. Any increase of lift coefficient on a wing also increases the strength of the vortex at the tip. Hence in a sideslip, the dihedral not only creates a strong rolling force to raise the into-slip wing, but a drag force also appears tending to yaw the aircraft towards the slip – a yaw one way, combined with a rolling force the other. Correction of the yaw depends mainly on the weathercock stability, which is why dihedral and fin areas are so closely coupled.
The yawing effect of dihedral in slipping or skidding is similar to the adverse yaw of ailerons, which will be discussed below (Chapter 13).