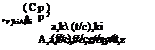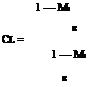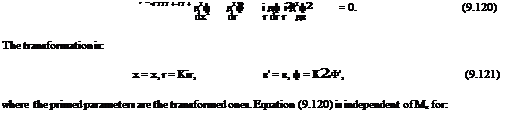Moving Disturbance
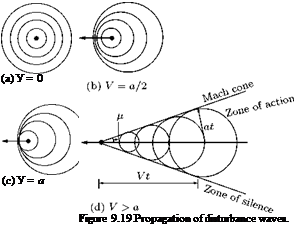
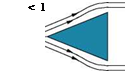
The presence of a small disturbance is felt throughout the field by means of disturbance waves traveling at the local velocity of sound relative to the medium. Let us examine the propagation of pressure disturbance created by a moving object shown in Figure 9.19. The propagation of disturbance waves created by an object moving with velocity V = 0, V = a/2, V = a and V > a is shown in Figures 9.19(a), (b), (c), (d), respectively. In a subsonic flow, the disturbance waves reach a stationary observer before the source of disturbance could reach him, as shown in Figures 9.19(a) and 9.19(b). But in supersonic flows it takes considerable amount of time for an observer to perceive the pressure disturbance, after the source has passed him. This is one of the fundamental differences between subsonic and supersonic flows. Therefore, in a subsonic flow the streamlines sense the presence of any obstacle in the flow field and adjust themselves well ahead of the obstacles and flow around it smoothly.
But in a supersonic flow, the streamlines feel the obstacle only when they hit it. The obstacle acts as a source and the streamlines deviate at the Mach cone as shown in Figure 9.19(d). That is in a supersonic flow the disturbance due to an obstacle is sudden and the flow behind the obstacle has to change abruptly.
 |
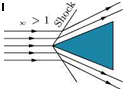 |
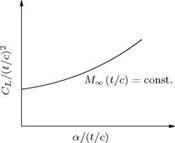
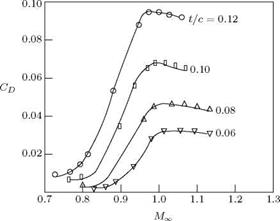
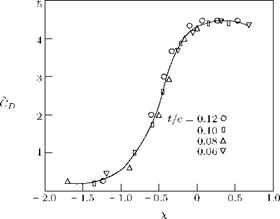
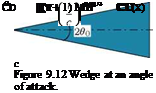
Flow around a wedge shown in Figures 9.20(a) and 9.20(b) illustrate the smooth and abrupt change in flow direction for subsonic and supersonic flow, respectively. For < 1, the flow direction changes smoothly and the pressure decreases with acceleration. For Mx > 1, there is a sudden change in flow direction at the body and the pressure increases downstream of the shock.
(a) Subsonic flow
In Figure 9.19(d), it is shown that for supersonic motion of an object there is a well-defined conical zone in the flow field with the object located at the nose of the cone and the disturbance created by the moving object is confined only to the field included inside the cone. The flow field zone outside the cone does not even feel the disturbance. For this reason, von Karman termed the region inside the cone as the zone of action and the region outside the cone as the zone of silence. The lines at which the pressure disturbance is concentrated and which generate the cone are called Mach waves or Mach lines. The angle between the Mach line and the direction of motion of the body is called the Mach angle p. From Figure 9.19(d), we have:
![]() at
at
sin a
1 Vt
that is:
From the disturbance waves propagation shown in Figure 9.19, we can infer the following features of the flow regimes:
• When the medium is incompressible (M = 0, Figure 9.19(a)) or when the speed of the moving disturbance is negligibly small compared to the local sound speed, the pressure pulse created by the disturbance spreads uniformly in all directions.
• When the disturbance source moves with a subsonic speed (M < 1, Figure 9.19(b)), the pressure disturbance is felt in all directions and at all points in space (neglecting viscous dissipation), but the pressure pattern is no longer symmetrical.
• For sonic velocity (M = 1, Figure 9.19(c)) the pressure pulse is at the boundary between subsonic and supersonic flow and the wave front is a plane.
• For supersonic speeds (M > 1, Figure 9.19(d)) the disturbance wave propagation phenomenon is totally different from those at subsonic speeds. All the pressure disturbances are included in a cone which has the disturbance source at its apex and the effect of the disturbance is not felt upstream of the disturbance source.
9.18.1 Small Disturbance
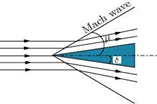 |
When the apex angle of wedge S is vanishingly small, the disturbances will be small and we can consider these disturbance waves to be identical to sound pulses. In such a case, the deviation of streamlines will be small and there will be infinitesimally small increase of pressure across the Mach cone shown in Figure 9.21.
9.18.2 Finite Disturbance
When the wedge angle S is finite the disturbances introduced are finite, then the wave is not called Mach wave but a shock or shock wave (see Figure 9.22). The angle of shock в is always smaller than the Mach angle. The deviation of the streamlines is finite and the pressure increase across a shock wave is finite.











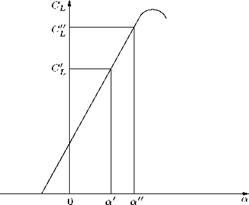
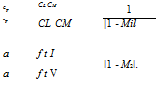 (9.118)
(9.118)