15.5.1.1 Incompressible Flow (M0 = 0)
Definition
In 2-D, the following aerodynamic coefficients C/, Cm, o, and Cd are defined as
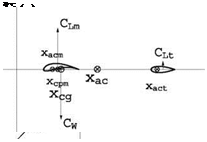
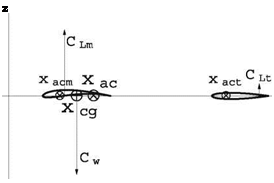 Fig. 15.13 Forces locations at take-off
Fig. 15.13 Forces locations at take-off
L’ _ M _ D
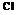 2 pU2c m’° 2 pU2c2’ d 2 pU2c
2 pU2c m’° 2 pU2c2’ d 2 pU2c
The expressions for Cl(a), Cm, o (a) and Cd for a symmetric profile are
Suction Force
See the sketch of the flat plate at incidence and of the force due to pressure integration and the suction force on Fig. 15.14.
The suction force coefficient can be obtained since the resulting drag coefficient must be zero. Hence
Cs — F’j ^—pU2c^ — Ci sin a = Ci a — 2na2
The thickness distribution contributes to recuperating the suction force by allowing the boundary layer to remain attached at the rounded leading edge, thanks to the elimination of the singularity at the sharp trailing edge of the plate.
Center of Pressure
Definition: the center of pressure is the point about which the moment of the aerodynamic forces is zero.
As seen in class, the center of pressure for a symmetric profile is at the quarter – chord. This is easily shown by using the transfer of moment formula
xcp Cm, o 1
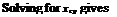
~ = cT = 4
For a symmetric profile, Cm, ac = 0, since the center of pressure coincides with the aerodynamic center (the formula for the value of Cm, ac is the same as that for
Cm, cp.
Computing Cm, o(a) from the Cm, ac, the change of moment formula gives
15.5.1.2 Supersonic Flow (Mo > 1, в = ^— 1)
A thin double wedge airfoil equips the fins of a missile cruising at Mach number M0 > 1 in a uniform atmosphere. The chord of the airfoil is c. The profile camber and thickness are:
d (x) = 0
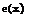 20x, 0 < x < c/2
20x, 0 < x < c/2
2в(е – x), c/2 < x < c
with z±(x) = d(x) ± e(x)/2.
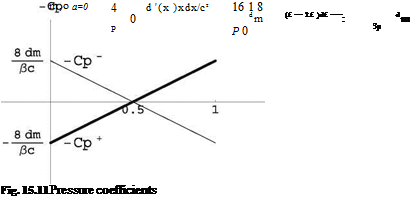
Pressure Distributions
C + and C – are the same at a = 0. One finds
C+ = C – = в в, 0 < x < c/2 C+ = C- = – вв, c/2 < x < c
See Fig. 15.15.
Lift Coefficient
The lift coefficient Ct (a) for all thin airfoils in supersonic flow is only a function of incidence
4
Ct (a) = a
P
|
Fig. 15.15 Pressure coefficients at a — 0
|
Drag Coefficient
The drag (wave drag) at zero incidence, (Cd)a=0, is
4 2 4 2
Cd (a) = в2 + a2
в в
Moment Coefficient
The zero incidence moment coefficient (Cm, o) 0 = 0 since it only depends on
camber.
Thus, the values of the coefficient Cm, o for the general case a = 0 is
Maximum Finess
The inverse of the finess, 1/f — Cd/Ci is
в2
1/f = + a
a
and the value of a that maximizes f satisfies
The solution is a = в (a =—в for negative lift). See Fig. 15.16 for remarkable waves (shocks, expansions).
![]() ideal ^1 + 2 ^^ = (CDt)ideal ^1 +
ideal ^1 + 2 ^^ = (CDt)ideal ^1 +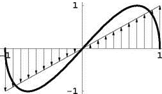











 Pressure Distribution and Global Coefficients
Pressure Distribution and Global Coefficients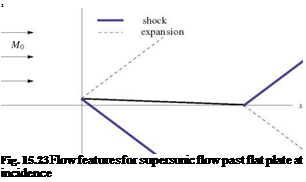
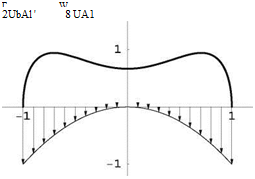
 Fig. 15.13 Forces locations at take-off
Fig. 15.13 Forces locations at take-off
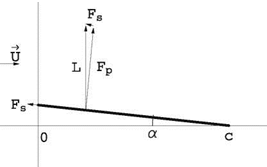

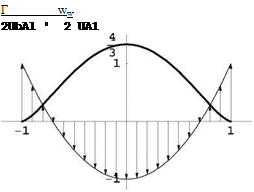 Fig. 15.12 Circulation and downwash for the wing with weak tip vortices
Fig. 15.12 Circulation and downwash for the wing with weak tip vortices