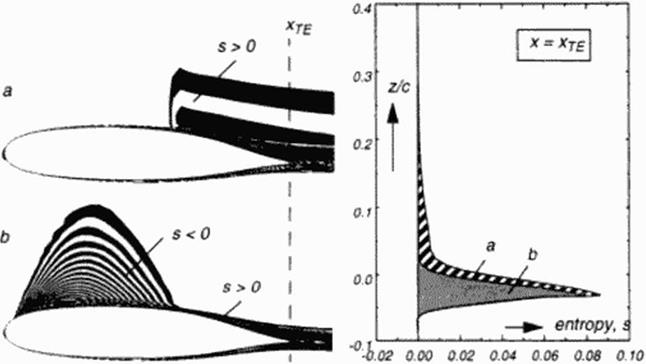
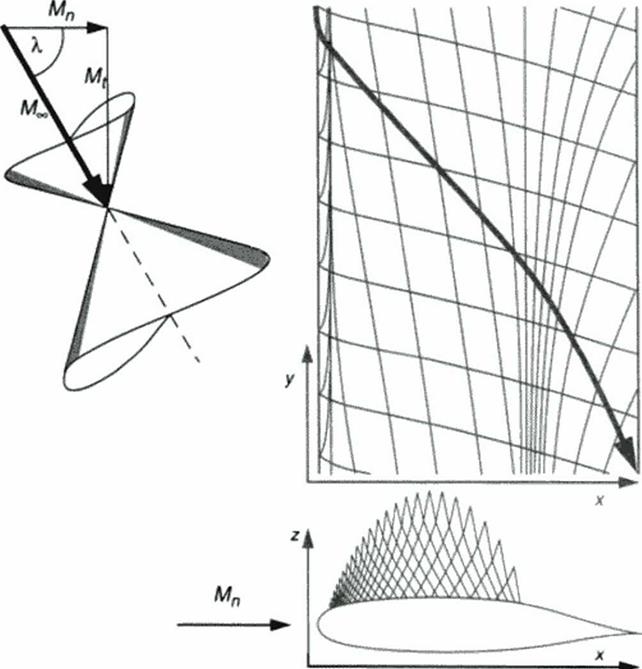
Near sonic Beltrami equations
The potential equation for a near-sonic plane or axisymmctric flow has a particularly elegant formulation in variables of state or in characteristic variables (for details see Ref (78)). illustrating the formal relationship between incompressible, transonic, plane and axially symmetric potential problems: characteristic equation and compatibility relation define a system of quasi-lincar first order differential equations
|
V, = YP’U,
|
(64)
|
|
V, = jYP‘U,
|
(65)
|
|
Xt = UP:Y,
|
(66)
|
|
X, = jUP‘Y,
|
(67)
|
Here the variables (X. Y) denote the physical space and (U, V) a n<»rmalizcd pair of velocity variables, namely the Prandil-Meyer function and the flow angle. Both pairs are
dependent variables in a workspace ts, t) “Rheograph plane” w hich is identical with characteristic variables in supersonic flow (j=l, U>0) and their analytical continuation beyond the sonic – line where j«-1, U < 0. Exponents pt and pj have a switch function: p, =0 denotes plane 2D flow, p, ■ 1 indicates axially symmetric flow; p> = 0 results in a simple mapping of linear subsonic or supersonic flow while PS = 1/3 switches to transonic flow. These equations include most of the flow models described by the pioneers in theoretical transomes. in closed analytical form or. for transonic axisyntmetric flow where a weak nonlinearity persists, in a numerically very suitable form
Equations (64M67) describe a large number of educational solutions which should be kept ‘alive’ as part of the knowledge base for transonic design and phenomena analysis. Because of (64)-(67) representing a system for quasi-conformal or characteristic mapping, solutions may be interpreted as transformations of geometries consisting of both the boundary conditions and ail details of the flow, appearing as an analog flow in the rheograph plane.
Applications of mapped problems to test cases for numerical methods
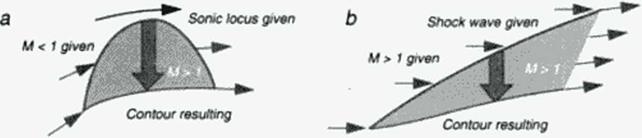
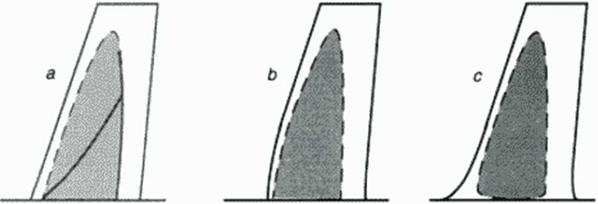
In the transonic and low supersonic Mach number regime, analytical soloutions describing the local or asymptotic behavior of shock waves in the flow arc of particular interest. Like the well – known logarithmic singularity (79) for the normal shock on a curved contour, there are other singular solutions for interaction of shock waves with solid boundaries or with flow phenomena near sonic conditions. Frequently, limiting cases between two known mathematical models have to be found. One such case is modelling a smooth transition from the mathematically well understood far field behavior of an airfoil in some flow * I to the appearance of a detached shock wave in front of it if the free stream М» slightly exceeds unity. This example was solved by a mapping to the rheograph plane (80).
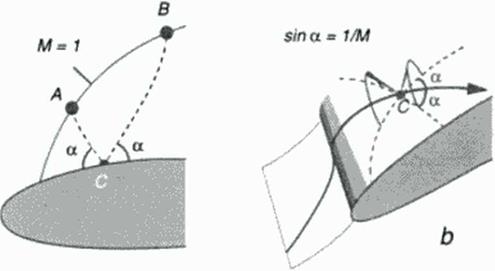
More recently a similar problem was solved by a local mapping of the 2D plane flow near the tip of a wedge ramp at precisely the attachment Mach number The limiting case
between a detached shock, normal to the flow axis, approaching the tip and an oblique shock attached to the wedge in supersonic flow (see the early experiments (81 ]>. had to be found. Figure I illustrates this limiting case both in the physical plane and mapped to the rheograph plane Results arc given in (82). here it’s just worth to mention that another, new logarithmic singularity at the wedge tip is found from simple mapping procedures.
The value of such local solutions for new and rerined computational methods in fluid dynamics is evident: known local flow models represent exact solutions and refinements should give information about needed efforts to obtain such solutions numerically to a desired degree of accuracy. The wedge problem serves as a test case for an unstructured grid Euler code [83) where local grid refinements allow for a sharpening of the shock waves occurring in the flow, see Figure 36.
|
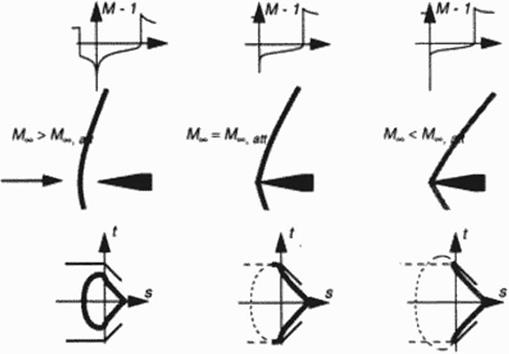
Figure 35 Understanding shock attachment / detachment to a wedge: Rheograph mapping and shock relations transform problem to an analog flow detail and conformal mapping case. From above: Aeial and surface Mach number distribution with changing wedge tip singularities; shock detachment, limiting case and shock attachment; shock polar growing and intersecting wedge boundary condition for increased Mach number.
|
|
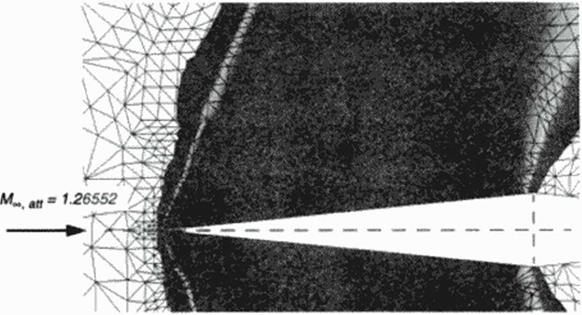
Figure 36 Numerical simulation of shock attaching to a wedge (slope 1:10 ). Simulation
with unstructured grid Euler code, grid adaptation near shock wave, isobars visualization
|